Skip to ContentGo to accessibility pageKeyboard shortcuts menu
OpenStax Logo
Intermediate Algebra

Review Exercises

Intermediate AlgebraReview Exercises
Menu
Table of contents
  1. Preface
  2. 1 Foundations
    1. Introduction
    2. 1.1 Use the Language of Algebra
    3. 1.2 Integers
    4. 1.3 Fractions
    5. 1.4 Decimals
    6. 1.5 Properties of Real Numbers
    7. Chapter Review
      1. Key Terms
      2. Key Concepts
    8. Exercises
      1. Review Exercises
      2. Practice Test
  3. 2 Solving Linear Equations
    1. Introduction
    2. 2.1 Use a General Strategy to Solve Linear Equations
    3. 2.2 Use a Problem Solving Strategy
    4. 2.3 Solve a Formula for a Specific Variable
    5. 2.4 Solve Mixture and Uniform Motion Applications
    6. 2.5 Solve Linear Inequalities
    7. 2.6 Solve Compound Inequalities
    8. 2.7 Solve Absolute Value Inequalities
    9. Chapter Review
      1. Key Terms
      2. Key Concepts
    10. Exercises
      1. Review Exercises
      2. Practice Test
  4. 3 Graphs and Functions
    1. Introduction
    2. 3.1 Graph Linear Equations in Two Variables
    3. 3.2 Slope of a Line
    4. 3.3 Find the Equation of a Line
    5. 3.4 Graph Linear Inequalities in Two Variables
    6. 3.5 Relations and Functions
    7. 3.6 Graphs of Functions
    8. Chapter Review
      1. Key Terms
      2. Key Concepts
    9. Exercises
      1. Review Exercises
      2. Practice Test
  5. 4 Systems of Linear Equations
    1. Introduction
    2. 4.1 Solve Systems of Linear Equations with Two Variables
    3. 4.2 Solve Applications with Systems of Equations
    4. 4.3 Solve Mixture Applications with Systems of Equations
    5. 4.4 Solve Systems of Equations with Three Variables
    6. 4.5 Solve Systems of Equations Using Matrices
    7. 4.6 Solve Systems of Equations Using Determinants
    8. 4.7 Graphing Systems of Linear Inequalities
    9. Chapter Review
      1. Key Terms
      2. Key Concepts
    10. Exercises
      1. Review Exercises
      2. Practice Test
  6. 5 Polynomials and Polynomial Functions
    1. Introduction
    2. 5.1 Add and Subtract Polynomials
    3. 5.2 Properties of Exponents and Scientific Notation
    4. 5.3 Multiply Polynomials
    5. 5.4 Dividing Polynomials
    6. Chapter Review
      1. Key Terms
      2. Key Concepts
    7. Exercises
      1. Review Exercises
      2. Practice Test
  7. 6 Factoring
    1. Introduction to Factoring
    2. 6.1 Greatest Common Factor and Factor by Grouping
    3. 6.2 Factor Trinomials
    4. 6.3 Factor Special Products
    5. 6.4 General Strategy for Factoring Polynomials
    6. 6.5 Polynomial Equations
    7. Chapter Review
      1. Key Terms
      2. Key Concepts
    8. Exercises
      1. Review Exercises
      2. Practice Test
  8. 7 Rational Expressions and Functions
    1. Introduction
    2. 7.1 Multiply and Divide Rational Expressions
    3. 7.2 Add and Subtract Rational Expressions
    4. 7.3 Simplify Complex Rational Expressions
    5. 7.4 Solve Rational Equations
    6. 7.5 Solve Applications with Rational Equations
    7. 7.6 Solve Rational Inequalities
    8. Chapter Review
      1. Key Terms
      2. Key Concepts
    9. Exercises
      1. Review Exercises
      2. Practice Test
  9. 8 Roots and Radicals
    1. Introduction
    2. 8.1 Simplify Expressions with Roots
    3. 8.2 Simplify Radical Expressions
    4. 8.3 Simplify Rational Exponents
    5. 8.4 Add, Subtract, and Multiply Radical Expressions
    6. 8.5 Divide Radical Expressions
    7. 8.6 Solve Radical Equations
    8. 8.7 Use Radicals in Functions
    9. 8.8 Use the Complex Number System
    10. Chapter Review
      1. Key Terms
      2. Key Concepts
    11. Exercises
      1. Review Exercises
      2. Practice Test
  10. 9 Quadratic Equations and Functions
    1. Introduction
    2. 9.1 Solve Quadratic Equations Using the Square Root Property
    3. 9.2 Solve Quadratic Equations by Completing the Square
    4. 9.3 Solve Quadratic Equations Using the Quadratic Formula
    5. 9.4 Solve Quadratic Equations in Quadratic Form
    6. 9.5 Solve Applications of Quadratic Equations
    7. 9.6 Graph Quadratic Functions Using Properties
    8. 9.7 Graph Quadratic Functions Using Transformations
    9. 9.8 Solve Quadratic Inequalities
    10. Chapter Review
      1. Key Terms
      2. Key Concepts
    11. Exercises
      1. Review Exercises
      2. Practice Test
  11. 10 Exponential and Logarithmic Functions
    1. Introduction
    2. 10.1 Finding Composite and Inverse Functions
    3. 10.2 Evaluate and Graph Exponential Functions
    4. 10.3 Evaluate and Graph Logarithmic Functions
    5. 10.4 Use the Properties of Logarithms
    6. 10.5 Solve Exponential and Logarithmic Equations
    7. Chapter Review
      1. Key Terms
      2. Key Concepts
    8. Exercises
      1. Review Exercises
      2. Practice Test
  12. 11 Conics
    1. Introduction
    2. 11.1 Distance and Midpoint Formulas; Circles
    3. 11.2 Parabolas
    4. 11.3 Ellipses
    5. 11.4 Hyperbolas
    6. 11.5 Solve Systems of Nonlinear Equations
    7. Chapter Review
      1. Key Terms
      2. Key Concepts
    8. Exercises
      1. Review Exercises
      2. Practice Test
  13. 12 Sequences, Series and Binomial Theorem
    1. Introduction
    2. 12.1 Sequences
    3. 12.2 Arithmetic Sequences
    4. 12.3 Geometric Sequences and Series
    5. 12.4 Binomial Theorem
    6. Chapter Review
      1. Key Terms
      2. Key Concepts
    7. Exercises
      1. Review Exercises
      2. Practice Test
  14. Answer Key
    1. Chapter 1
    2. Chapter 2
    3. Chapter 3
    4. Chapter 4
    5. Chapter 5
    6. Chapter 6
    7. Chapter 7
    8. Chapter 8
    9. Chapter 9
    10. Chapter 10
    11. Chapter 11
    12. Chapter 12
  15. Index

Review Exercises

Distance and Midpoint Formulas; Circles

Use the Distance Formula

In the following exercises, find the distance between the points. Round to the nearest tenth if needed.

244.

(−5,1)(−5,1) and (−1,4)(−1,4)

245.

(−2,5)(−2,5) and (1,5)(1,5)

246.

(8,2)(8,2) and (−7,−3)(−7,−3)

247.

(1,−4)(1,−4) and (5,−5)(5,−5)

Use the Midpoint Formula

In the following exercises, find the midpoint of the line segments whose endpoints are given.

248.

(−2,−6)(−2,−6) and (−4,−2)(−4,−2)

249.

(3,7)(3,7) and (5,1)(5,1)

250.

(−8,−10)(−8,−10) and (9,5)(9,5)

251.

(−3,2)(−3,2) and (6,−9)(6,−9)

Write the Equation of a Circle in Standard Form

In the following exercises, write the standard form of the equation of the circle with the given information.

252.

radius is 15 and center is (0,0)(0,0)

253.

radius is 77 and center is (0,0)(0,0)

254.

radius is 9 and center is (−3,5)(−3,5)

255.

radius is 7 and center is (−2,−5)(−2,−5)

256.

center is (3,6)(3,6) and a point on the circle is (3,−2)(3,−2)

257.

center is (2,2)(2,2) and a point on the circle is (4,4)(4,4)

Graph a Circle

In the following exercises, ⓐ find the center and radius, then ⓑ graph each circle.

258.

2 x 2 + 2 y 2 = 450 2 x 2 + 2 y 2 = 450

259.

3 x 2 + 3 y 2 = 432 3 x 2 + 3 y 2 = 432

260.

( x + 3 ) 2 + ( y − 5 ) 2 = 81 ( x + 3 ) 2 + ( y − 5 ) 2 = 81

261.

( x + 2 ) 2 + ( y + 5 ) 2 = 49 ( x + 2 ) 2 + ( y + 5 ) 2 = 49

262.

x 2 + y 2 − 6 x − 12 y − 19 = 0 x 2 + y 2 − 6 x − 12 y − 19 = 0

263.

x 2 + y 2 − 4 y − 60 = 0 x 2 + y 2 − 4 y − 60 = 0

Parabolas

Graph Vertical Parabolas

In the following exercises, graph each equation by using its properties.

264.

y = x 2 + 4 x − 3 y = x 2 + 4 x − 3

265.

y = 2 x 2 + 10 x + 7 y = 2 x 2 + 10 x + 7

266.

y = −6 x 2 + 12 x − 1 y = −6 x 2 + 12 x − 1

267.

y = − x 2 + 10 x y = − x 2 + 10 x

In the following exercises, ⓐ write the equation in standard form, then ⓑ use properties of the standard form to graph the equation.

268.

y = x 2 + 4 x + 7 y = x 2 + 4 x + 7

269.

y = 2 x 2 − 4 x − 2 y = 2 x 2 − 4 x − 2

270.

y = −3 x 2 − 18 x − 29 y = −3 x 2 − 18 x − 29

271.

y = − x 2 + 12 x − 35 y = − x 2 + 12 x − 35

Graph Horizontal Parabolas

In the following exercises, graph each equation by using its properties.

272.

x = 2 y 2 x = 2 y 2

273.

x = 2 y 2 + 4 y + 6 x = 2 y 2 + 4 y + 6

274.

x = − y 2 + 2 y − 4 x = − y 2 + 2 y − 4

275.

x = −3 y 2 x = −3 y 2

In the following exercises, ⓐ write the equation in standard form, then ⓑ use properties of the standard form to graph the equation.

276.

x = 4 y 2 + 8 y x = 4 y 2 + 8 y

277.

x = y 2 + 4 y + 5 x = y 2 + 4 y + 5

278.

x = − y 2 − 6 y − 7 x = − y 2 − 6 y − 7

279.

x = −2 y 2 + 4 y x = −2 y 2 + 4 y

Solve Applications with Parabolas

In the following exercises, create the equation of the parabolic arch formed in the foundation of the bridge shown. Give the answer in standard form.

280.
The figure shows a parabolic arch formed in the foundation of the bridge. The arch is 5 feet high and 20 feet wide.
281.
The figure shows a parabolic arch formed in the foundation of the bridge. The arch is 25 feet high and 30 feet wide.
Ellipses

Graph an Ellipse with Center at the Origin

In the following exercises, graph each ellipse.

282.

x 2 36 + y 2 25 = 1 x 2 36 + y 2 25 = 1

283.

x 2 4 + y 2 81 = 1 x 2 4 + y 2 81 = 1

284.

49 x 2 + 64 y 2 = 3136 49 x 2 + 64 y 2 = 3136

285.

9 x 2 + y 2 = 9 9 x 2 + y 2 = 9

Find the Equation of an Ellipse with Center at the Origin

In the following exercises, find the equation of the ellipse shown in the graph.

286.
The figure shows an ellipse graphed on the x y coordinate plane. The ellipse has a center at (0, 0), a horizontal major axis, vertices at (plus or minus 10, 0), and co-vertices at (0, plus or minus 4).
287.
The figure shows an ellipse graphed on the x y coordinate plane. The ellipse has a center at (0, 0), a vertical major axis, vertices at (0, plus or minus 8), and co-vertices at (plus or minus 6, 0).

Graph an Ellipse with Center Not at the Origin

In the following exercises, graph each ellipse.

288.

( x − 1 ) 2 25 + ( y − 6 ) 2 4 = 1 ( x − 1 ) 2 25 + ( y − 6 ) 2 4 = 1

289.

( x + 4 ) 2 16 + ( y + 1 ) 2 9 = 1 ( x + 4 ) 2 16 + ( y + 1 ) 2 9 = 1

290.

( x − 5 ) 2 16 + ( y + 3 ) 2 36 = 1 ( x − 5 ) 2 16 + ( y + 3 ) 2 36 = 1

291.

( x + 3 ) 2 9 + ( y − 2 ) 2 25 = 1 ( x + 3 ) 2 9 + ( y − 2 ) 2 25 = 1

In the following exercises, ⓐ write the equation in standard form and ⓑ graph.

292.

x 2 + y 2 + 12 x + 40 y + 120 = 0 x 2 + y 2 + 12 x + 40 y + 120 = 0

293.

25 x 2 + 4 y 2 − 150 x − 56 y + 321 = 0 25 x 2 + 4 y 2 − 150 x − 56 y + 321 = 0

294.

25 x 2 + 4 y 2 + 150 x + 125 = 0 25 x 2 + 4 y 2 + 150 x + 125 = 0

295.

4 x 2 + 9 y 2 − 126 x + 405 = 0 4 x 2 + 9 y 2 − 126 x + 405 = 0

Solve Applications with Ellipses

In the following exercises, write the equation of the ellipse described.

296.

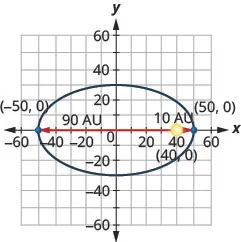
A comet moves in an elliptical orbit around a sun. The closest the comet gets to the sun is approximately 10 AU and the furthest is approximately 90 AU. The sun is one of the foci of the elliptical orbit. Letting the ellipse center at the origin and labeling the axes in AU, the orbit will look like the figure below. Use the graph to write an equation for the elliptical orbit of the comet.

The figure shows a model of an elliptical orbit around the sun on the x y coordinate plane. The ellipse has a center at (0, 0), a horizontal major axis, vertices marked at (plus or minus 50, 0), the sun marked as a foci and labeled (50, 0), the closest distance the comet is from the sun marked as 10 A U, and the farthest a comet is from the sun marked as 90 A U.
Hyperbolas

Graph a Hyperbola with Center at (0,0)(0,0)

In the following exercises, graph.

297.

x 2 25 − y 2 9 = 1 x 2 25 − y 2 9 = 1

298.

y 2 49 − x 2 16 = 1 y 2 49 − x 2 16 = 1

299.

9 y 2 − 16 x 2 = 144 9 y 2 − 16 x 2 = 144

300.

16 x 2 − 4 y 2 = 64 16 x 2 − 4 y 2 = 64

Graph a Hyperbola with Center at (h,k)(h,k)

In the following exercises, graph.

301.

( x + 1 ) 2 4 − ( y + 1 ) 2 9 = 1 ( x + 1 ) 2 4 − ( y + 1 ) 2 9 = 1

302.

( x − 2 ) 2 4 − ( y − 3 ) 2 16 = 1 ( x − 2 ) 2 4 − ( y − 3 ) 2 16 = 1

303.

( y + 2 ) 2 9 − ( x + 1 ) 2 9 = 1 ( y + 2 ) 2 9 − ( x + 1 ) 2 9 = 1

304.

( y − 1 ) 2 25 − ( x − 2 ) 2 9 = 1 ( y − 1 ) 2 25 − ( x − 2 ) 2 9 = 1

In the following exercises, ⓐ write the equation in standard form and ⓑ graph.

305.

4 x 2 − 16 y 2 + 8 x + 96 y − 204 = 0 4 x 2 − 16 y 2 + 8 x + 96 y − 204 = 0

306.

16 x 2 − 4 y 2 − 64 x − 24 y − 36 = 0 16 x 2 − 4 y 2 − 64 x − 24 y − 36 = 0

307.

4 y 2 − 16 x 2 + 32 x − 8 y − 76 = 0 4 y 2 − 16 x 2 + 32 x − 8 y − 76 = 0

308.

36 y 2 − 16 x 2 − 96 x + 216 y − 396 = 0 36 y 2 − 16 x 2 − 96 x + 216 y − 396 = 0

Identify the Graph of each Equation as a Circle, Parabola, Ellipse, or Hyperbola

In the following exercises, identify the type of graph.

309.


ⓐ 16y2−9x2−36x−96y−36=016y2−9x2−36x−96y−36=0
ⓑ x2+y2−4x+10y−7=0x2+y2−4x+10y−7=0
ⓒ y=x2−2x+3y=x2−2x+3
ⓓ 25x2+9y2=22525x2+9y2=225

310.


ⓐ x2+y2+4x−10y+25=0x2+y2+4x−10y+25=0
ⓑ y2−x2−4y+2x−6=0y2−x2−4y+2x−6=0
ⓒ x=−y2−2y+3x=−y2−2y+3
ⓓ 16x2+9y2=14416x2+9y2=144

Solve Systems of Nonlinear Equations

Solve a System of Nonlinear Equations Using Graphing

In the following exercises, solve the system of equations by using graphing.

311.

{ 3 x 2 − y = 0 y = 2 x − 1 { 3 x 2 − y = 0 y = 2 x − 1

312.

{ y = x 2 − 4 y = x − 4 { y = x 2 − 4 y = x − 4

313.

{ x 2 + y 2 = 169 x = 12 { x 2 + y 2 = 169 x = 12

314.

{ x 2 + y 2 = 25 y = −5 { x 2 + y 2 = 25 y = −5

Solve a System of Nonlinear Equations Using Substitution

In the following exercises, solve the system of equations by using substitution.

315.

{ y = x 2 + 3 y = −2 x + 2 { y = x 2 + 3 y = −2 x + 2

316.

{ x 2 + y 2 = 4 x − y = 4 { x 2 + y 2 = 4 x − y = 4

317.

{ 9 x 2 + 4 y 2 = 36 y − x = 5 { 9 x 2 + 4 y 2 = 36 y − x = 5

318.

{ x 2 + 4 y 2 = 4 2 x − y = 1 { x 2 + 4 y 2 = 4 2 x − y = 1

Solve a System of Nonlinear Equations Using Elimination

In the following exercises, solve the system of equations by using elimination.

319.

{ x 2 + y 2 = 16 x 2 − 2 y − 1 = 0 { x 2 + y 2 = 16 x 2 − 2 y − 1 = 0

320.

{ x 2 − y 2 = 5 − 2 x 2 − 3 y 2 = −30 { x 2 − y 2 = 5 − 2 x 2 − 3 y 2 = −30

321.

{ 4 x 2 + 9 y 2 = 36 3 y 2 − 4 x = 12 { 4 x 2 + 9 y 2 = 36 3 y 2 − 4 x = 12

322.

{ x 2 + y 2 = 14 x 2 − y 2 = 16 { x 2 + y 2 = 14 x 2 − y 2 = 16

Use a System of Nonlinear Equations to Solve Applications

In the following exercises, solve the problem using a system of equations.

323.

The sum of the squares of two numbers is 25. The difference of the numbers is 1. Find the numbers.

324.

The difference of the squares of two numbers is 45. The difference of the square of the first number and twice the square of the second number is 9. Find the numbers.

325.

The perimeter of a rectangle is 58 meters and its area is 210 square meters. Find the length and width of the rectangle.

326.

Colton purchased a larger microwave for his kitchen. The diagonal of the front of the microwave measures 34 inches. The front also has an area of 480 square inches. What are the length and width of the microwave?

PreviousNext
Order a print copy

As an Amazon Associate we earn from qualifying purchases.

Citation/Attribution

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Attribution information
  • If you are redistributing all or part of this book in a print format, then you must include on every physical page the following attribution:
    Access for free at https://openstax.org/books/intermediate-algebra/pages/1-introduction
  • If you are redistributing all or part of this book in a digital format, then you must include on every digital page view the following attribution:
    Access for free at https://openstax.org/books/intermediate-algebra/pages/1-introduction
Citation information

© Feb 9, 2022 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.