Skip to ContentGo to accessibility pageKeyboard shortcuts menu
OpenStax Logo
Contemporary Mathematics

Chapter Review

Contemporary MathematicsChapter Review
Table of contents
  1. Preface
  2. 1 Sets
    1. Introduction
    2. 1.1 Basic Set Concepts
    3. 1.2 Subsets
    4. 1.3 Understanding Venn Diagrams
    5. 1.4 Set Operations with Two Sets
    6. 1.5 Set Operations with Three Sets
    7. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  3. 2 Logic
    1. Introduction
    2. 2.1 Statements and Quantifiers
    3. 2.2 Compound Statements
    4. 2.3 Constructing Truth Tables
    5. 2.4 Truth Tables for the Conditional and Biconditional
    6. 2.5 Equivalent Statements
    7. 2.6 De Morgan’s Laws
    8. 2.7 Logical Arguments
    9. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Projects
      5. Chapter Review
      6. Chapter Test
  4. 3 Real Number Systems and Number Theory
    1. Introduction
    2. 3.1 Prime and Composite Numbers
    3. 3.2 The Integers
    4. 3.3 Order of Operations
    5. 3.4 Rational Numbers
    6. 3.5 Irrational Numbers
    7. 3.6 Real Numbers
    8. 3.7 Clock Arithmetic
    9. 3.8 Exponents
    10. 3.9 Scientific Notation
    11. 3.10 Arithmetic Sequences
    12. 3.11 Geometric Sequences
    13. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  5. 4 Number Representation and Calculation
    1. Introduction
    2. 4.1 Hindu-Arabic Positional System
    3. 4.2 Early Numeration Systems
    4. 4.3 Converting with Base Systems
    5. 4.4 Addition and Subtraction in Base Systems
    6. 4.5 Multiplication and Division in Base Systems
    7. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Projects
      5. Chapter Review
      6. Chapter Test
  6. 5 Algebra
    1. Introduction
    2. 5.1 Algebraic Expressions
    3. 5.2 Linear Equations in One Variable with Applications
    4. 5.3 Linear Inequalities in One Variable with Applications
    5. 5.4 Ratios and Proportions
    6. 5.5 Graphing Linear Equations and Inequalities
    7. 5.6 Quadratic Equations with Two Variables with Applications
    8. 5.7 Functions
    9. 5.8 Graphing Functions
    10. 5.9 Systems of Linear Equations in Two Variables
    11. 5.10 Systems of Linear Inequalities in Two Variables
    12. 5.11 Linear Programming
    13. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  7. 6 Money Management
    1. Introduction
    2. 6.1 Understanding Percent
    3. 6.2 Discounts, Markups, and Sales Tax
    4. 6.3 Simple Interest
    5. 6.4 Compound Interest
    6. 6.5 Making a Personal Budget
    7. 6.6 Methods of Savings
    8. 6.7 Investments
    9. 6.8 The Basics of Loans
    10. 6.9 Understanding Student Loans
    11. 6.10 Credit Cards
    12. 6.11 Buying or Leasing a Car
    13. 6.12 Renting and Homeownership
    14. 6.13 Income Tax
    15. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  8. 7 Probability
    1. Introduction
    2. 7.1 The Multiplication Rule for Counting
    3. 7.2 Permutations
    4. 7.3 Combinations
    5. 7.4 Tree Diagrams, Tables, and Outcomes
    6. 7.5 Basic Concepts of Probability
    7. 7.6 Probability with Permutations and Combinations
    8. 7.7 What Are the Odds?
    9. 7.8 The Addition Rule for Probability
    10. 7.9 Conditional Probability and the Multiplication Rule
    11. 7.10 The Binomial Distribution
    12. 7.11 Expected Value
    13. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Formula Review
      4. Projects
      5. Chapter Review
      6. Chapter Test
  9. 8 Statistics
    1. Introduction
    2. 8.1 Gathering and Organizing Data
    3. 8.2 Visualizing Data
    4. 8.3 Mean, Median and Mode
    5. 8.4 Range and Standard Deviation
    6. 8.5 Percentiles
    7. 8.6 The Normal Distribution
    8. 8.7 Applications of the Normal Distribution
    9. 8.8 Scatter Plots, Correlation, and Regression Lines
    10. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  10. 9 Metric Measurement
    1. Introduction
    2. 9.1 The Metric System
    3. 9.2 Measuring Area
    4. 9.3 Measuring Volume
    5. 9.4 Measuring Weight
    6. 9.5 Measuring Temperature
    7. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  11. 10 Geometry
    1. Introduction
    2. 10.1 Points, Lines, and Planes
    3. 10.2 Angles
    4. 10.3 Triangles
    5. 10.4 Polygons, Perimeter, and Circumference
    6. 10.5 Tessellations
    7. 10.6 Area
    8. 10.7 Volume and Surface Area
    9. 10.8 Right Triangle Trigonometry
    10. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  12. 11 Voting and Apportionment
    1. Introduction
    2. 11.1 Voting Methods
    3. 11.2 Fairness in Voting Methods
    4. 11.3 Standard Divisors, Standard Quotas, and the Apportionment Problem
    5. 11.4 Apportionment Methods
    6. 11.5 Fairness in Apportionment Methods
    7. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  13. 12 Graph Theory
    1. Introduction
    2. 12.1 Graph Basics
    3. 12.2 Graph Structures
    4. 12.3 Comparing Graphs
    5. 12.4 Navigating Graphs
    6. 12.5 Euler Circuits
    7. 12.6 Euler Trails
    8. 12.7 Hamilton Cycles
    9. 12.8 Hamilton Paths
    10. 12.9 Traveling Salesperson Problem
    11. 12.10 Trees
    12. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Videos
      4. Formula Review
      5. Projects
      6. Chapter Review
      7. Chapter Test
  14. 13 Math and...
    1. Introduction
    2. 13.1 Math and Art
    3. 13.2 Math and the Environment
    4. 13.3 Math and Medicine
    5. 13.4 Math and Music
    6. 13.5 Math and Sports
    7. Chapter Summary
      1. Key Terms
      2. Key Concepts
      3. Formula Review
      4. Projects
      5. Chapter Review
      6. Chapter Test
  15. A | Co-Req Appendix: Integer Powers of 10
  16. Answer Key
    1. Chapter 1
    2. Chapter 2
    3. Chapter 3
    4. Chapter 4
    5. Chapter 5
    6. Chapter 6
    7. Chapter 7
    8. Chapter 8
    9. Chapter 9
    10. Chapter 10
    11. Chapter 11
    12. Chapter 12
    13. Chapter 13
  17. Index

Chapter Review

Points, Lines, and Planes
Use the figure shown for the following exercises.
A line with four points, A, B, C, and D marked on it.
1 .
Find B C → ∪ C D → .
2 .
Find A C ¯ ∩ B C ¯ .
3 .
Name the points in the set B D → ∩ C A ← .
Angles
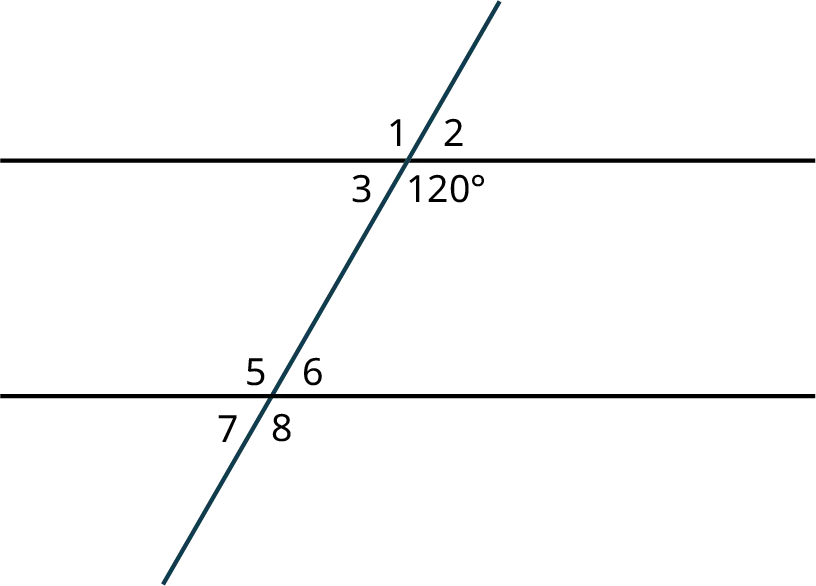
4 .
Given that l 1 and l 2 are parallel lines, solve the angle measurements for all the angles in the figure shown.
Two parallel horizontal lines are intersected by a transversal. The transversal makes four angles labeled 1, 2, 3, and 120 degrees with the line at the top. The transversal makes four angles numbered 5, 6, 7, and 8 with the line at the bottom. 1, 2, 7, and 8 are exterior angles. 3, 120 degrees, 5, and 6 are interior angles.
5 .
Find the measure of the vertical angles in the figure shown.
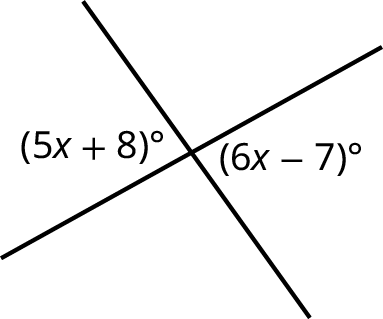
Two lines intersect each other forming four angles. One set of opposite angles is labeled (5 x plus 8) degrees and (6 x minus 7) degrees.
6 .
Classify the angle as acute, right, or obtuse: 79 ∘ .
7 .
Use the given figure to solve for the angles.
A horizontal line and a vertical line intersect each other forming a right angle. A line originates from the intersection of the horizontal and vertical lines. This line makes an angle, 3 x plus 1 degrees with the horizontal line. This line makes an angle, 7 x plus 12 degrees with the vertical line.
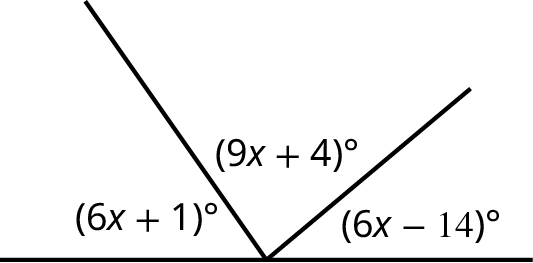
8 .
Use the given figure to solve for the angle measurements.
A horizontal line with two lines originating from its center. The first ray makes an angle, 6 x minus 14 degrees with the horizontal line. The angle formed between the two rays is labeled 9 x plus 4 degrees. The second ray makes an angle, 6 x plus 1 degrees with the horizontal line.
Triangles
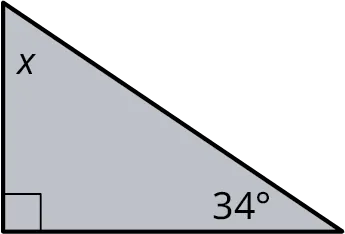
9 .
Use the given figure to find the measure of the unknown angles.
A right triangle with its interior angles marked x, 90 degrees, and 34 degrees.
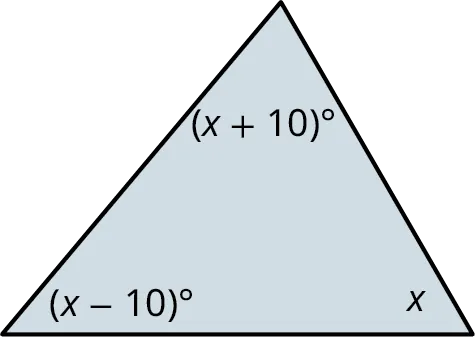
10 .
Use algebra to find the measure of the angles in the figure shown.
A triangle with its interior angles marked (x plus 10) degrees, (x minus 10) degrees, and x.
11 .
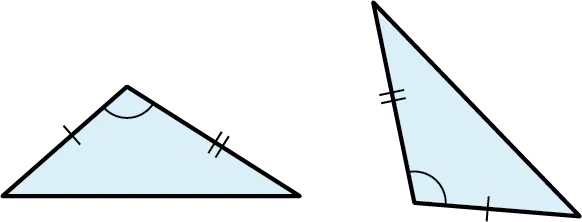
The two triangles shown are congruent by what theorem?
Two triangles. The left side of the first triangle and the bottom side of the second triangle are congruent. The left side of the second triangle and the right side of the first triangle are congruent. The top angle of the first triangle and the bottom-left angle of the second triangle are congruent.
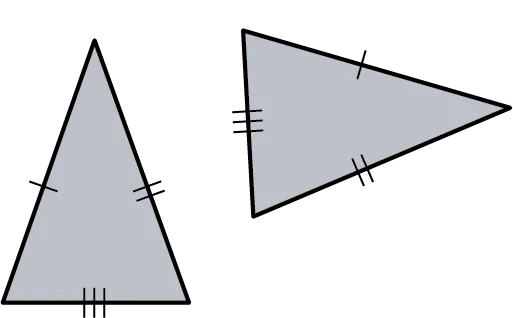
12 .
The two triangles shown are congruent by what theorem?
Two triangles. The left side of the first triangle and the top side of the second triangle are congruent. The right side of the first triangle and the bottom side of the second triangle are congruent. The bottom side of the first triangle and the left side of the second triangle are congruent.
13 .
The two triangles shown are congruent by what theorem?
Two triangles. The left side of the first triangle and the top side of the second triangle are congruent. The top-right angle of the first triangle and the top-left of the second triangle are congruent. The bottom-right angle of the first triangle and the bottom-left angle of the second triangle are congruent.
14 .
Are the two figures shown similar?
Two smiley faces. The first one is smaller and the second one is bigger.
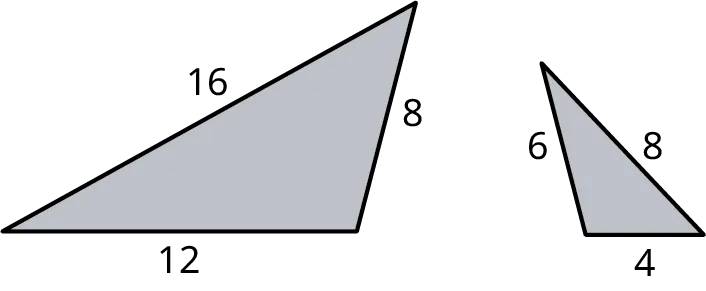
15 .
Are the two triangles shown similar?
Two triangles. The sides of the first triangle are marked 16, 8, and 12. The sides of the second triangle are marked 6, 8, and 4.
16 .
Find the scaling factor of the two similar triangles in the given figure.
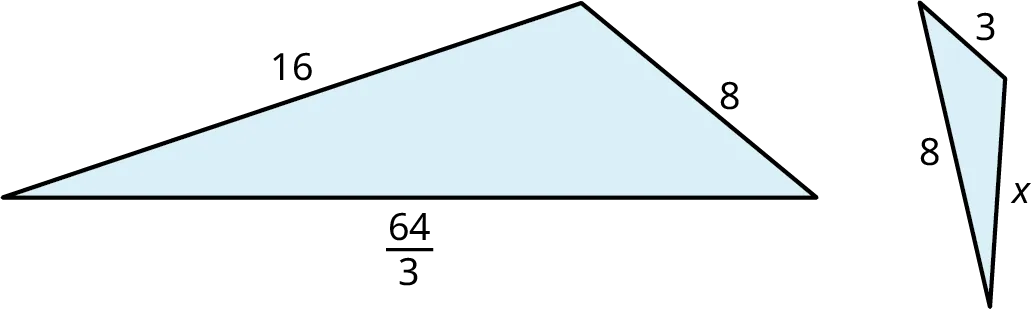
Two triangles. The sides of the first triangle are marked 16, 8, and 64 over 3. The sides of the second triangle are marked 3, 8, and x.
17 .
Find the length of x in the figure shown.
Polygons, Perimeter, and Circumference
Identify the polygons.
18 .
A polygon with six equal sides.
19 .
A polygon with four equal sides and no right angles. Two diagonal lines run through the polygon.
20 .
A polygon with eight equal sides.
21 .
Find the perimeter of a regular hexagon with side length 5 cm.
22 .
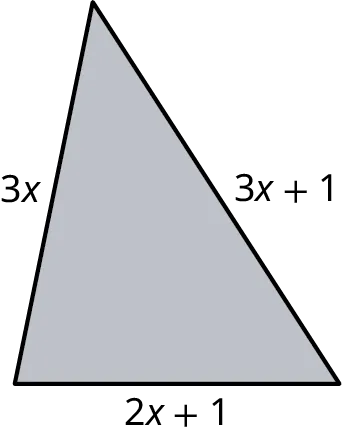
The perimeter of a triangle is 18 in the given figure. Find the length of the sides.
A triangle with its sides marked 3 x, 3 x plus 1, and 2 x plus 1.
23 .
What is the measure of an interior angle of a regular heptagon?
24 .
What is the measure of an interior angle of a regular octagon?
25 .
Calculate the measure of each interior angle in the figure shown.
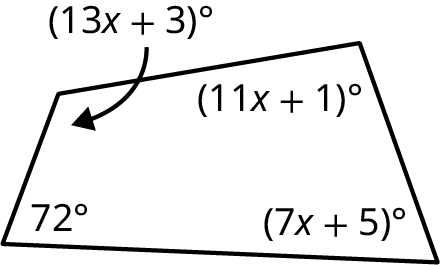
A quadrilateral with its angles marked (13 x plus 3) degrees, (11 x plus 1) degrees, 72 degrees, and (7 x plus 5) degrees.
26 .
Find the measure of an exterior angle of a regular pentagon.
27 .
What is the sum of the measures of the exterior angles of a regular heptagon?
28 .
Find the circumference of a circle with radius 3.2 cm.
29 .
Find the diameter of a circle with a circumference of 35.6 in.
Tessellations
30 .
In what field of mathematics does the topic of tessellations belong?
31 .
Tessellations can have no _______or _________.
32 .
What type of transformation moves an object over horizontally by some number of units?
33 .
What type of transformation results in a mirror image of the shape?
34 .
Which regular polygons will tessellate the plane by themselves?
35 .
The sum of the interior angles of the shapes meeting at a vertex is equal to how many degrees?
36 .
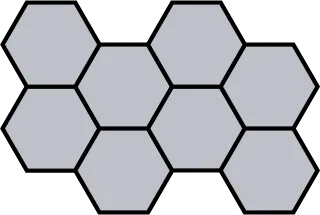
How would you name this tessellation?
A tessellation pattern is made up of 8 hexagons.
Area
37 .
What is the area of a triangle with a base equal to 5 cm and a height equal to 12 cm?
38 .
If the area of a triangle equals 24  cm 2 and the base equals 8, what is the height?
39 .
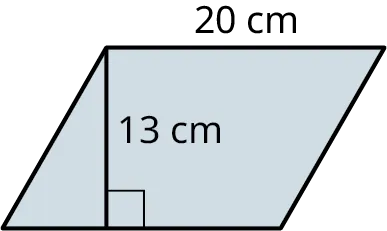
Find the area of the parallelogram shown.
A parallelogram with its length marked 20 centimeters and height marked 13 centimeters.
40 .
If the area of a trapezoid equals 45 2  cm 2 , b 1 = 3  cm , and the height equals h = 5  cm , find the length of b 2 .
41 .
Find the area of an octagon if the apothem equals 10 cm and the side length is 12 cm.
42 .
The area of a circle is 50.3  in 2 . What is the radius?
43 .
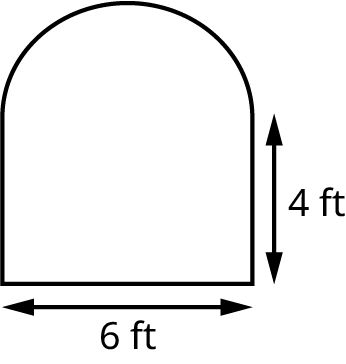
You want to install a tinted protective shield on this window. How many square feet do you order?
A figure shows a semicircle placed on top of a rectangle. The length and width of the rectangle are 6 feet and 4 feet.
44 .
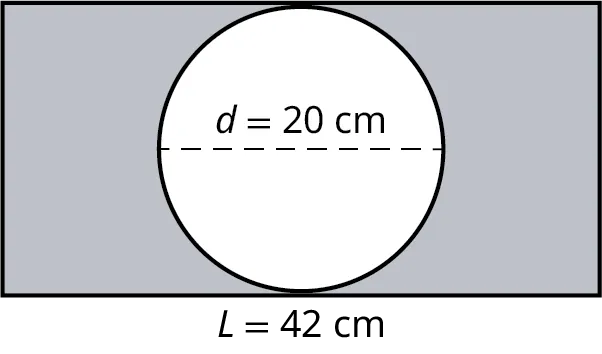
Find the area of the shaded region in the given figure.

A circle is enclosed within a rectangle. The length of the rectangle is marked L equals 42 centimeters. The diameter of the circle is marked 20 centimeters. The circle touches the top and bottom sides of the rectangle.
Volume and Surface Area
45 .
Find the surface area of a hexagonal prism with side length 5 cm, apothem 3.1 cm, and height 15 cm.
A triangular prism has an equilateral base and side lengths of 15 in, triangle height of 10 in, and prism length of 25 in.
46 .
Find the surface area of the triangular prism.
47 .
Find the volume of the triangular prism.
48 .
Find the volume of a right cylinder with radius equal to 1.5 cm and height equal to 5 cm.
A right cylinder has a radius of 6 cm and a height of 10 cm.
49 .
Find the surface area of the right cylinder.
50 .
Find the volume of the right cylinder.
Right Triangle Trigonometry
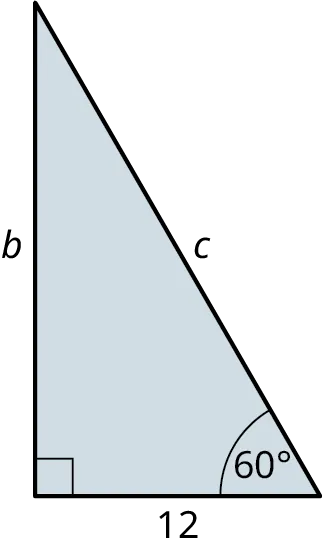
51 .
Find the lengths of the missing sides in the figure shown.
A right triangle. The legs are marked 6 and 12. The hypotenuse is marked c. The angle formed by the horizontal leg and hypotenuse is marked 60 degrees.
52 .
Find the measure of the unknown side and angle.
A right triangle. The legs are marked 3.44 and 7. The hypotenuse is marked c. The angle formed by the horizontal leg and hypotenuse is marked theta.
PreviousNext
Order a print copy

As an Amazon Associate we earn from qualifying purchases.

Citation/Attribution

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Attribution information
  • If you are redistributing all or part of this book in a print format, then you must include on every physical page the following attribution:
    Access for free at https://openstax.org/books/contemporary-mathematics/pages/1-introduction
  • If you are redistributing all or part of this book in a digital format, then you must include on every digital page view the following attribution:
    Access for free at https://openstax.org/books/contemporary-mathematics/pages/1-introduction
Citation information

© Apr 17, 2023 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.