Donna Kirk

Chapter Test

1 .

Perform the indicated operation for the expression:

(3{x^2} + 2x + 1) - ({x^2} - 2 + 2) + x(3x + 11)

2 .

Solve the linear equations using properties of equations:

x(x - 2) + 5 = 9x + x(x - 6)

3 .

It costs 55 cents for a stamp. Construct a linear equation and solve how much it cost to buy 50 stamps.

4 .

Solve the formula

A = \frac{{\left( {{b_1}\, + \,{b_2}} \right)}}{2}h

for

h

.

5 .

Solve the inequality

-2x < -6

, graph the solution on the number line, and write the solution in interval notation.

6 .

Construct a linear inequality to solve the application: Bella wants to buy a round of shakes for her friends. It will cost $4.75 per shake, including tip and tax. Her budget is $50. What is the maximum number of friends Bella can buy shakes for?

7 .

Manneken Pis is a famous statue in Brussels, Belgium. It is 24 inches tall and weighs 37.5 pounds. The average man is 69 inches tall and weighs 198 pounds. Is Manneken Pis proportional to the average male?

8 .

Graph

y = \frac{1}{4}x + 1

by plotting points.

9 .

Graph the linear inequality:

y \ge 2x + 7

10 .

Graph and list the solutions to the quadratic equation

{x^2} - 36 = 0

.

11 .

Solve

{x^2} - 49 = 0

by factoring.

12 .

Solve

{x^2} + 5x + 2 = 0

using the quadratic formula.

13 .

Evaluate the function

f(x) = -3x + 21

at the values

f (-2)

,

f (-1)

,

f (0)

,

f(1)

, and

f (2)

.

14 .

Use the vertical line test to determine if the given graph represents a function. A function is plotted on an x y coordinate plane. The x and y axes range from negative 10 to 10, in increments of 1. The function passes through the points, (negative 8, negative 1), (negative 4.5, 1), (negative 1.5, negative 1), (1.5, 1), (4.5, negative 1), and (7.5, 1). Note: all values are approximate.

A function is plotted on an x y coordinate plane. The x and y axes range from negative 10 to 10, in increments of 1. The function passes through the points, (negative 8, negative 1), (negative 4.5, 1), (negative 1.5, negative 1), (1.5, 1), (4.5, negative 1), and (7.5, 1). Note: all values are approximate.

15 .

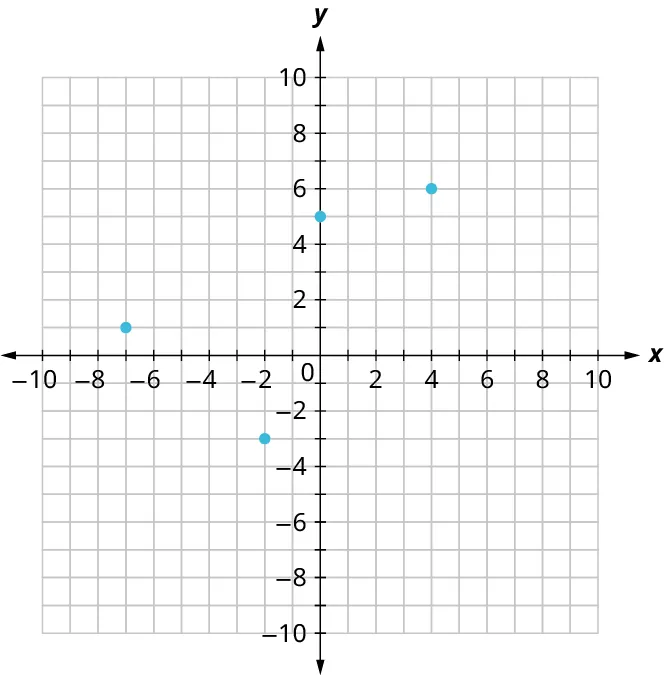
Use the graph shown to find the domain and the range. Four points are plotted on an x y coordinate plane. The x and y axes range from negative 10 to 10, in increments of 1. The points are plotted at the following coordinates: (negative 7, 1), (negative 2, negative 3), (0, 5), and (4, 6).

Four points are plotted on an x y coordinate plane. The x and y axes range from negative 10 to 10, in increments of 1. The points are plotted at the following coordinates: (negative 7, 1), (negative 2, negative 3), (0, 5), and (4, 6).

16 .

Graph

2y = x - 4

using the intercepts.

17 .

Use the slope formula to find the slope of the line between (1, 4) and (3, 5).

18 .

Identify the slope and

y

-intercept of

y - \frac{2}{3}x = 1

.

19 .

Graph the line of

y = \frac{2}{3}x + 1

using its slope and

y

-intercept.

The equation

C = 4.50 + 15p

, models the cost of visiting the Cat Café in San Diego for one hour.

C

, in dollars, is the total cost and the cost per person,

p

, is $15 plus a $4.50 reservation fee.

20 .

Find the payment for two people.

21 .

Find the payment for five people.

22 .

Interpret the slope and

C

-intercept of the equation.

23 .

Graph the equation.

24 .

Solve the system of equations by graphing.

\left\{ {\begin{array}{*{20}{l}}{y - 3x = 3}\\{y = - 6x + 12}\end{array}} \right.

25 .

Solve the system of equations by substitution.

\left\{ {\begin{array}{*{20}{l}}{y = 5x - 5}\\{ - 3x + y = - 3}\end{array}} \right.

26 .

Solve the systems of equations by elimination.

\left\{ {\begin{array}{*{20}{l}}{y = \frac{1}{3}x - 6}\\{y = x - 3}\end{array}} \right.

27 .

Anna goes to the concession stand at a movie theater. She buys 5 popcorns and 4 large sodas and pays a total of $60. During intermission, Isabelle goes to the concession stand. She buys 1 popcorn and 2 large sodas and pays a total of $18. What is the cost of one popcorn, and the cost of one large soda?

28 .

Solve the systems of linear equations by graphing.

\left\{ {\begin{array}{*{20}{l}}{y > \frac{1}{3}x - 1}\\{y < x - 3}\end{array}} \right.

Juliette is selling fresh lemonade and cupcakes. She sells a cup of lemonade for $2 and a cupcake for $3. She needs to make at least $100 to donate to the local cat sanctuary. She needs to sell at least 20 cups of lemonade.

29 .

Write a system of inequalities to model this situation.

30 .

Graph the system.

31 .

Could she sell 30 cups of lemonade and 10 cupcakes and make $100?

32 .

Could she sell 20 cups of lemonade and 30 cupcakes and make $100?

A toy maker makes exactly two toys out of wood; the Box (

x

) and the Bat (

y

). He makes $5 per Box and $6 per Bat. Each Box requires 30 ounces of wood, and each Bat requires 45 ounces of wood. Today the toy maker has 270 ounces of wood available. The toy maker also only makes 8 wooden toys per day. To maximize profit, how many of each wooden toy should the toy maker make?

33 .

Find the objective function.

34 .

Write the constraints as a system of inequalities.

35 .

Graph of the system of inequalities.

36 .

Find the value of the objective function at each corner point of the graphed region.

37 .

To maximize profit, how many of each toy should the toymaker make?