Quadratic formula
If then
| Triangle of base and height |
Area |
|
| Circle of radius |
Circumference |
Area |
| Sphere of radius |
Surface area |
Volume |
| Cylinder of radius and height |
Area of curved surface |
Volume |
Table
E1
Geometry
Trigonometry
Trigonometric Identities
Triangles
- Law of sines:
- Law of cosines:
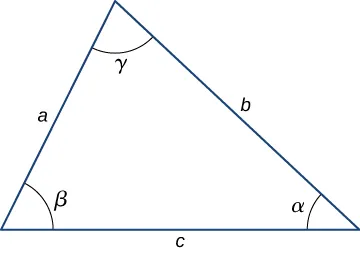
- Pythagorean theorem:

Series expansions
- Binomial theorem:
Derivatives
Integrals
