Practice Test
For the following exercises, sketch the graph of each function for two full periods. Determine the amplitude, the period, and the equation for the midline.
1.
2.
3.
4.
5.
6.
7.
8.
9.
10.
11.
12.
13.
For the following exercises, determine the amplitude, period, and midline of the graph, and then find a formula for the function.
14.
Give in terms of a sine function.
![A graph of two periods of a sine function, graphed from -2 to 2. Range is [-6,-2], period is 2, and amplitude is 2.](/apps/image-cdn/v1/f=webp/apps/archive/20250916.165151/resources/0ca7251e757afc8749770cff689a429ccb582f51)
15.
Give in terms of a sine function.
![A graph of two periods of a sine function, graphed over -2 to 2. Range is [-2,2], period is 2, and amplitude is 2.](/apps/image-cdn/v1/f=webp/apps/archive/20250916.165151/resources/f3c9a5750eeddbdb679ce9dc4b78a1d54df4f7ab)
16.
Give in terms of a tangent function.
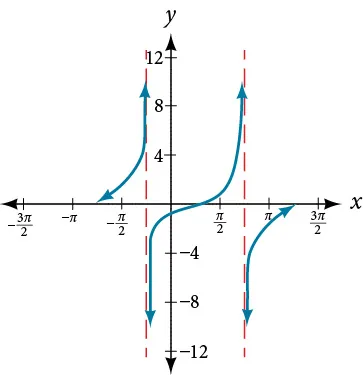
For the following exercises, find the amplitude, period, phase shift, and midline.
17.
18.
19.
The outside temperature over the course of a day can be modeled as a sinusoidal function. Suppose you know the temperature is 68°F at midnight and the high and low temperatures during the day are 80°F and 56°F, respectively. Assuming is the number of hours since midnight, find a function for the temperature, in terms of
20.
Water is pumped into a storage bin and empties according to a periodic rate. The depth of the water is 3 feet at its lowest at 2:00 a.m. and 71 feet at its highest, which occurs every 5 hours. Write a cosine function that models the depth of the water as a function of time, and then graph the function for one period.
For the following exercises, find the period and horizontal shift of each function.
21.
22.
23.
Write the equation for the graph in Figure 1 in terms of the secant function and give the period and phase shift.
24.
If find
25.
If find
For the following exercises, graph the functions on the specified window and answer the questions.
26.
Graph on the viewing window by Approximate the graph’s period.
27.
Graph on the following domains in and Suppose this function models sound waves. Why would these views look so different?
28.
Graph on and explain any observations.
For the following exercises, let
29.
What is the largest possible value for
30.
What is the smallest possible value for
31.
Where is the function increasing on the interval
For the following exercises, find and graph one period of the periodic function with the given amplitude, period, and phase shift.
32.
Sine curve with amplitude 3, period and phase shift
33.
Cosine curve with amplitude 2, period and phase shift
For the following exercises, graph the function. Describe the graph and, wherever applicable, any periodic behavior, amplitude, asymptotes, or undefined points.
34.
35.
For the following exercises, find the exact value.
36.
37.
38.
39.
40.
41.
42.
43.
For the following exercises, suppose
Evaluate the following expressions.
46.
Given Figure 2, find the measure of angle to three decimal places. Answer in radians.
For the following exercises, determine whether the equation is true or false.
47.
48.
49.
The grade of a road is 7%. This means that for every horizontal distance of 100 feet on the road, the vertical rise is 7 feet. Find the angle the road makes with the horizontal in radians.
