Lynn Marecek; MaryAnne Anthony-Smith

9.3 Add and Subtract Square Roots

Learning Objectives

By the end of this section, you will be able to:

Add and subtract like square roots
Add and subtract square roots that need simplification

Be Prepared 9.3

Before you get started, take this readiness quiz.

Add: ⓐ $3 x + 9 x$ ⓑ $5 m + 5 n$ .
If you missed this problem, review Example 1.24.
Simplify: $\sqrt{50 x^{3}}$ .
If you missed this problem, review Example 9.16.

We know that we must follow the order of operations to simplify expressions with square roots. The radical is a grouping symbol, so we work inside the radical first. We simplify $\sqrt{2 + 7}$ in this way:

$\begin{matrix} \sqrt{2 + 7} \\ Add inside the radical. & \sqrt{9} \\ Simplify. & 3 \end{matrix}$

So if we have to add $\sqrt{2} + \sqrt{7}$ , we must not combine them into one radical.

\sqrt{2} + \sqrt{7} \neq \sqrt{2 + 7}

Trying to add square roots with different radicands is like trying to add unlike terms.

$\begin{matrix} But, just like we can add & x + x, & we can add & \sqrt{3} + \sqrt{3} . \\ x + x = 2 x & \sqrt{3} + \sqrt{3} = 2 \sqrt{3} \end{matrix}$

Adding square roots with the same radicand is just like adding like terms. We call square roots with the same radicand like square roots to remind us they work the same as like terms.

Like Square Roots

Square roots with the same radicand are called like square roots.

We add and subtract like square roots in the same way we add and subtract like terms. We know that $3 x + 8 x$ is $11 x$ . Similarly we add $3 \sqrt{x} + 8 \sqrt{x}$ and the result is $11 \sqrt{x} .$

Add and Subtract Like Square Roots

Think about adding like terms with variables as you do the next few examples. When you have like radicands, you just add or subtract the coefficients. When the radicands are not like, you cannot combine the terms.

Example 9.29

Simplify: $2 \sqrt{2} - 7 \sqrt{2}$ .

Solution

$\begin{matrix} 2 \sqrt{2} - 7 \sqrt{2} \\ \begin{matrix} Since the radicals are like, we subtract the \\ coefficients. \end{matrix} & −5 \sqrt{2} \end{matrix}$

Try It 9.57

Simplify: $8 \sqrt{2} - 9 \sqrt{2}$ .

Try It 9.58

Simplify: $5 \sqrt{3} - 9 \sqrt{3}$ .

Example 9.30

Simplify: $3 \sqrt{y} + 4 \sqrt{y}$ .

Solution

$\begin{matrix} 3 \sqrt{y} + 4 \sqrt{y} \\ \begin{matrix} Since the radicals are like, we add the \\ coefficients. \end{matrix} & 7 \sqrt{y} \end{matrix}$

Try It 9.59

Simplify: $2 \sqrt{x} + 7 \sqrt{x}$ .

Try It 9.60

Simplify: $5 \sqrt{u} + 3 \sqrt{u}$ .

Example 9.31

Simplify: $4 \sqrt{x} - 2 \sqrt{y}$ .

Solution

$\begin{matrix} 4 \sqrt{x} - 2 \sqrt{y} \\ \begin{matrix} Since the radicals are not like, we cannot \\ subtract them. We leave the expression as is. \end{matrix} & 4 \sqrt{x} - 2 \sqrt{y} \end{matrix}$

Try It 9.61

Simplify: $7 \sqrt{p} - 6 \sqrt{q}$ .

Try It 9.62

Simplify: $6 \sqrt{a} - 3 \sqrt{b}$ .

Example 9.32

Simplify: $5 \sqrt{13} + 4 \sqrt{13} + 2 \sqrt{13}$ .

Solution

$\begin{matrix} 5 \sqrt{13} + 4 \sqrt{13} + 2 \sqrt{13} \\ \begin{matrix} Since the radicals are like, we add the \\ coefficients. \end{matrix} & 11 \sqrt{13} \end{matrix}$

Try It 9.63

Simplify: $4 \sqrt{11} + 2 \sqrt{11} + 3 \sqrt{11}$ .

Try It 9.64

Simplify: $6 \sqrt{10} + 2 \sqrt{10} + 3 \sqrt{10}$ .

Example 9.33

Simplify: $2 \sqrt{6} - 6 \sqrt{6} + 3 \sqrt{3}$ .

Solution

$\begin{matrix} 2 \sqrt{6} - 6 \sqrt{6} + 3 \sqrt{3} \\ \begin{matrix} Since the first two radicals are like, we \\ subtract their coefficients. \end{matrix} & - 4 \sqrt{6} + 3 \sqrt{3} \end{matrix}$

Try It 9.65

Simplify: $5 \sqrt{5} - 4 \sqrt{5} + 2 \sqrt{6}$ .

Try It 9.66

Simplify: $3 \sqrt{7} - 8 \sqrt{7} + 2 \sqrt{5}$ .

Example 9.34

Simplify: $2 \sqrt{5 n} - 6 \sqrt{5 n} + 4 \sqrt{5 n}$ .

Solution

$\begin{matrix} 2 \sqrt{5 n} - 6 \sqrt{5 n} + 4 \sqrt{5 n} \\ Since the radicals are like, we combine them. & 0 \sqrt{5 n} \\ Simplify. & 0 \end{matrix}$

Try It 9.67

Simplify: $\sqrt{7 x} - 7 \sqrt{7 x} + 4 \sqrt{7 x}$ .

Try It 9.68

Simplify: $4 \sqrt{3 y} - 7 \sqrt{3 y} + 2 \sqrt{3 y}$ .

When radicals contain more than one variable, as long as all the variables and their exponents are identical, the radicals are like.

Example 9.35

Simplify: $\sqrt{3 x y} + 5 \sqrt{3 x y} - 4 \sqrt{3 x y}$ .

Solution

$\begin{matrix} \sqrt{3 x y} + 5 \sqrt{3 x y} - 4 \sqrt{3 x y} \\ Since the radicals are like, we combine them. & 2 \sqrt{3 x y} \end{matrix}$

Try It 9.69

Simplify: $\sqrt{5 x y} + 4 \sqrt{5 x y} - 7 \sqrt{5 x y}$ .

Try It 9.70

Simplify: $3 \sqrt{7 m n} + \sqrt{7 m n} - 4 \sqrt{7 m n}$ .

Add and Subtract Square Roots that Need Simplification

Remember that we always simplify square roots by removing the largest perfect-square factor. Sometimes when we have to add or subtract square roots that do not appear to have like radicals, we find like radicals after simplifying the square roots.

Example 9.36

Simplify: $\sqrt{20} + 3 \sqrt{5}$ .

Solution

$\begin{matrix} \sqrt{20} + 3 \sqrt{5} \\ Simplify the radicals, when possible. & \sqrt{4} \cdot \sqrt{5} + 3 \sqrt{5} \\ 2 \sqrt{5} + 3 \sqrt{5} \\ Combine the like radicals. & 5 \sqrt{5} \end{matrix}$

Try It 9.71

Simplify: $\sqrt{18} + 6 \sqrt{2}$ .

Try It 9.72

Simplify: $\sqrt{27} + 4 \sqrt{3}$ .

Example 9.37

Simplify: $\sqrt{48} - \sqrt{75}$ .

Solution

$\begin{matrix} \sqrt{48} - \sqrt{75} \\ Simplify the radicals. & \sqrt{16} \cdot \sqrt{3} - \sqrt{25} \cdot \sqrt{3} \\ 4 \sqrt{3} - 5 \sqrt{3} \\ Combine the like radicals. & − \sqrt{3} \end{matrix}$

Try It 9.73

Simplify: $\sqrt{32} - \sqrt{18}$ .

Try It 9.74

Simplify: $\sqrt{20} - \sqrt{45}$ .

Just like we use the Associative Property of Multiplication to simplify $5 (3 x)$ and get $15 x$ , we can simplify $5 (3 \sqrt{x})$ and get $15 \sqrt{x}$ . We will use the Associative Property to do this in the next example.

Example 9.38

Simplify: $5 \sqrt{18} - 2 \sqrt{8}$ .

Solution

$\begin{matrix} 5 \sqrt{18} - 2 \sqrt{8} \\ Simplify the radicals. & 5 \cdot \sqrt{9} \cdot \sqrt{2} - 2 \cdot \sqrt{4} \cdot \sqrt{2} \\ 5 \cdot 3 \cdot \sqrt{2} - 2 \cdot 2 \cdot \sqrt{2} \\ 15 \sqrt{2} - 4 \sqrt{2} \\ Combine the like radicals. & 11 \sqrt{2} \end{matrix}$

Try It 9.75

Simplify: $4 \sqrt{27} - 3 \sqrt{12}$ .

Try It 9.76

Simplify: $3 \sqrt{20} - 7 \sqrt{45}$ .

Example 9.39

Simplify: $\frac{3}{4} \sqrt{192} - \frac{5}{6} \sqrt{108}$ .

Solution

$\begin{matrix} \frac{3}{4} \sqrt{192} - \frac{5}{6} \sqrt{108} \\ Simplify the radicals. & \frac{3}{4} \sqrt{64} \cdot \sqrt{3} - \frac{5}{6} \sqrt{36} \cdot \sqrt{3} \\ \frac{3}{4} \cdot 8 \cdot \sqrt{3} - \frac{5}{6} \cdot 6 \cdot \sqrt{3} \\ 6 \sqrt{3} - 5 \sqrt{3} \\ Combine the like radicals. & \sqrt{3} \end{matrix}$

Try It 9.77

Simplify: $\frac{2}{3} \sqrt{108} - \frac{5}{7} \sqrt{147}$ .

Try It 9.78

Simplify: $\frac{3}{5} \sqrt{200} - \frac{3}{4} \sqrt{128}$ .

Example 9.40

Simplify: $\frac{2}{3} \sqrt{48} - \frac{3}{4} \sqrt{12}$ .

Solution

$\begin{matrix} \frac{2}{3} \sqrt{48} - \frac{3}{4} \sqrt{12} \\ Simplify the radicals. & \frac{2}{3} \sqrt{16} \cdot \sqrt{3} - \frac{3}{4} \sqrt{4} \cdot \sqrt{3} \\ \frac{2}{3} \cdot 4 \cdot \sqrt{3} - \frac{3}{4} \cdot 2 \cdot \sqrt{3} \\ \frac{8}{3} \sqrt{3} - \frac{3}{2} \sqrt{3} \\ \begin{matrix} Find a common denominator to subtract the \\ coefficients of the like radicals. \end{matrix} & \frac{16}{6} \sqrt{3} - \frac{9}{6} \sqrt{3} \\ Simplify. & \frac{7}{6} \sqrt{3} \end{matrix}$

Try It 9.79

Simplify: $\frac{2}{5} \sqrt{32} - \frac{1}{3} \sqrt{8}$ .

Try It 9.80

Simplify: $\frac{1}{3} \sqrt{80} - \frac{1}{4} \sqrt{125}$ .

In the next example, we will remove constant and variable factors from the square roots.

Example 9.41

Simplify: $\sqrt{18 n^{5}} - \sqrt{32 n^{5}}$ .

Solution

$\begin{matrix} \sqrt{18 n^{5}} - \sqrt{32 n^{5}} \\ Simplify the radicals. & \sqrt{9 n^{4}} \cdot \sqrt{2 n} - \sqrt{16 n^{4}} \cdot \sqrt{2 n} \\ 3 n^{2} \sqrt{2 n} - 4 n^{2} \sqrt{2 n} \\ Combine the like radicals. & − n^{2} \sqrt{2 n} \end{matrix}$

Try It 9.81

Simplify: $\sqrt{32 m^{7}} - \sqrt{50 m^{7}}$ .

Try It 9.82

Simplify: $\sqrt{27 p^{3}} - \sqrt{48 p^{3}}$ .

Example 9.42

Simplify: $9 \sqrt{50 m^{2}} - 6 \sqrt{48 m^{2}}$ .

Solution

$\begin{matrix} 9 \sqrt{50 m^{2}} - 6 \sqrt{48 m^{2}} \\ Simplify the radicals. & 9 \sqrt{25 m^{2}} \cdot \sqrt{2} - 6 \sqrt{16 m^{2}} \cdot \sqrt{3} \\ 9 \cdot 5 m \cdot \sqrt{2} - 6 \cdot 4 m \cdot \sqrt{3} \\ 45 m \sqrt{2} - 24 m \sqrt{3} \\ \begin{matrix} The radicals are not like and so cannot be \\ combined. \end{matrix} \end{matrix}$

Try It 9.83

Simplify: $5 \sqrt{32 x^{2}} - 3 \sqrt{48 x^{2}}$ .

Try It 9.84

Simplify: $7 \sqrt{48 y^{2}} - 4 \sqrt{72 y^{2}}$ .

Example 9.43

Simplify: $2 \sqrt{8 x^{2}} - 5 x \sqrt{32} + 5 \sqrt{18 x^{2}}$ .

Solution

$\begin{matrix} 2 \sqrt{8 x^{2}} - 5 x \sqrt{32} + 5 \sqrt{18 x^{2}} \\ Simplify the radicals. & 2 \sqrt{4 x^{2}} \cdot \sqrt{2} - 5 x \sqrt{16} \cdot \sqrt{2} + 5 \sqrt{9 x^{2}} \cdot \sqrt{2} \\ 2 \cdot 2 x \cdot \sqrt{2} - 5 x \cdot 4 \cdot \sqrt{2} + 5 \cdot 3 x \cdot \sqrt{2} \\ 4 x \sqrt{2} - 20 x \sqrt{2} + 15 x \sqrt{2} \\ Combine the like radicals. & − x \sqrt{2} \end{matrix}$

Try It 9.85

Simplify: $3 \sqrt{12 x^{2}} - 2 x \sqrt{48} + 4 \sqrt{27 x^{2}}$ .

Try It 9.86

Simplify: $3 \sqrt{18 x^{2}} - 6 x \sqrt{32} + 2 \sqrt{50 x^{2}}$ .

Media

Access this online resource for additional instruction and practice with the adding and subtracting square roots.

Adding/Subtracting Square Roots

Section 9.3 Exercises

Practice Makes Perfect

Add and Subtract Like Square Roots

In the following exercises, simplify.

145.

$8 \sqrt{2} - 5 \sqrt{2}$

146.

$7 \sqrt{2} - 3 \sqrt{2}$

147.

$3 \sqrt{5} + 6 \sqrt{5}$

148.

$4 \sqrt{5} + 8 \sqrt{5}$

149.

$9 \sqrt{7} - 10 \sqrt{7}$

150.

$11 \sqrt{7} - 12 \sqrt{7}$

151.

$7 \sqrt{y} + 2 \sqrt{y}$

152.

$9 \sqrt{n} + 3 \sqrt{n}$

153.

$\sqrt{a} - 4 \sqrt{a}$

154.

$\sqrt{b} - 6 \sqrt{b}$

155.

$5 \sqrt{c} + 2 \sqrt{c}$

156.

$7 \sqrt{d} + 2 \sqrt{d}$

157.

$8 \sqrt{a} - 2 \sqrt{b}$

158.

$5 \sqrt{c} - 3 \sqrt{d}$

159.

$5 \sqrt{m} + \sqrt{n}$

160.

$\sqrt{n} + 3 \sqrt{p}$

161.

$8 \sqrt{7} + 2 \sqrt{7} + 3 \sqrt{7}$

162.

$6 \sqrt{5} + 3 \sqrt{5} + \sqrt{5}$

163.

$3 \sqrt{11} + 2 \sqrt{11} - 8 \sqrt{11}$

164.

$2 \sqrt{15} + 5 \sqrt{15} - 9 \sqrt{15}$

165.

$3 \sqrt{3} - 8 \sqrt{3} + 7 \sqrt{5}$

166.

$5 \sqrt{7} - 8 \sqrt{7} + 6 \sqrt{3}$

167.

$6 \sqrt{2} + 2 \sqrt{2} - 3 \sqrt{5}$

168.

$7 \sqrt{5} + \sqrt{5} - 8 \sqrt{10}$

169.

$3 \sqrt{2 a} - 4 \sqrt{2 a} + 5 \sqrt{2 a}$

170.

$\sqrt{11 b} - 5 \sqrt{11 b} + 3 \sqrt{11 b}$

171.

$8 \sqrt{3 c} + 2 \sqrt{3 c} - 9 \sqrt{3 c}$

172.

$3 \sqrt{5 d} + 8 \sqrt{5 d} - 11 \sqrt{5 d}$

173.

$5 \sqrt{3 a b} + \sqrt{3 a b} - 2 \sqrt{3 a b}$

174.

$8 \sqrt{11 c d} + 5 \sqrt{11 c d} - 9 \sqrt{11 c d}$

175.

$2 \sqrt{p q} - 5 \sqrt{p q} + 4 \sqrt{p q}$

176.

$11 \sqrt{2 r s} - 9 \sqrt{2 r s} + 3 \sqrt{2 r s}$

Add and Subtract Square Roots that Need Simplification

In the following exercises, simplify.

177.

$\sqrt{50} + 4 \sqrt{2}$

178.

$\sqrt{48} + 2 \sqrt{3}$

179.

$\sqrt{80} - 3 \sqrt{5}$

180.

$\sqrt{28} - 4 \sqrt{7}$

181.

$\sqrt{27} - \sqrt{75}$

182.

$\sqrt{72} - \sqrt{98}$

183.

$\sqrt{48} + \sqrt{27}$

184.

$\sqrt{45} + \sqrt{80}$

185.

$2 \sqrt{50} - 3 \sqrt{72}$

186.

$3 \sqrt{98} - \sqrt{128}$

187.

$2 \sqrt{12} + 3 \sqrt{48}$

188.

$4 \sqrt{75} + 2 \sqrt{108}$

189.

$\frac{2}{3} \sqrt{72} + \frac{1}{5} \sqrt{50}$

190.

$\frac{2}{5} \sqrt{75} + \frac{3}{4} \sqrt{48}$

191.

$\frac{1}{2} \sqrt{20} - \frac{2}{3} \sqrt{45}$

192.

$\frac{2}{3} \sqrt{54} - \frac{3}{4} \sqrt{96}$

193.

$\frac{1}{6} \sqrt{27} - \frac{3}{8} \sqrt{48}$

194.

$\frac{1}{8} \sqrt{32} - \frac{1}{10} \sqrt{50}$

195.

$\frac{1}{4} \sqrt{98} - \frac{1}{3} \sqrt{128}$

196.

$\frac{1}{3} \sqrt{24} + \frac{1}{4} \sqrt{54}$

197.

$\sqrt{72 a^{5}} - \sqrt{50 a^{5}}$

198.

$\sqrt{48 b^{5}} - \sqrt{75 b^{5}}$

199.

$\sqrt{80 c^{7}} - \sqrt{20 c^{7}}$

200.

$\sqrt{96 d^{9}} - \sqrt{24 d^{9}}$

201.

$9 \sqrt{80 p^{4}} - 6 \sqrt{98 p^{4}}$

202.

$8 \sqrt{72 q^{6}} - 3 \sqrt{75 q^{6}}$

203.

$2 \sqrt{50 r^{8}} + 4 \sqrt{54 r^{8}}$

204.

$5 \sqrt{27 s^{6}} + 2 \sqrt{20 s^{6}}$

205.

$3 \sqrt{20 x^{2}} - 4 \sqrt{45 x^{2}} + 5 x \sqrt{80}$

206.

$2 \sqrt{28 x^{2}} - \sqrt{63 x^{2}} + 6 x \sqrt{7}$

207.

$3 \sqrt{128 y^{2}} + 4 y \sqrt{162} - 8 \sqrt{98 y^{2}}$

208.

$3 \sqrt{75 y^{2}} + 8 y \sqrt{48} - \sqrt{300 y^{2}}$

Mixed Practice

209.

$2 \sqrt{8} + 6 \sqrt{8} - 5 \sqrt{8}$

210.

$\frac{2}{3} \sqrt{27} + \frac{3}{4} \sqrt{48}$

211.

$\sqrt{175 k^{4}} - \sqrt{63 k^{4}}$

212.

$\frac{5}{6} \sqrt{162} + \frac{3}{16} \sqrt{128}$

213.

$2 \sqrt{363} - 2 \sqrt{300}$

214.

$\sqrt{150} + 4 \sqrt{6}$

215.

$9 \sqrt{2} - 8 \sqrt{2}$

216.

$5 \sqrt{x} - 8 \sqrt{y}$

217.

$8 \sqrt{13} - 4 \sqrt{13} - 3 \sqrt{13}$

218.

$5 \sqrt{12 c^{4}} - 3 \sqrt{27 c^{6}}$

219.

$\sqrt{80 a^{5}} - \sqrt{45 a^{5}}$

220.

$\frac{3}{5} \sqrt{75} - \frac{1}{4} \sqrt{48}$

221.

$21 \sqrt{19} - 2 \sqrt{19}$

222.

$\sqrt{500} + \sqrt{405}$

223.

$\frac{5}{6} \sqrt{27} + \frac{5}{8} \sqrt{48}$

224.

$11 \sqrt{11} - 10 \sqrt{11}$

225.

$\sqrt{75} - \sqrt{108}$

226.

$2 \sqrt{98} - 4 \sqrt{72}$

227.

$4 \sqrt{24 x^{2}} - \sqrt{54 x^{2}} + 3 x \sqrt{6}$

228.

$8 \sqrt{80 y^{6}} - 6 \sqrt{48 y^{6}}$

Everyday Math

229.

A decorator decides to use square tiles as an accent strip in the design of a new shower, but she wants to rotate the tiles to look like diamonds. She will use 9 large tiles that measure 8 inches on a side and 8 small tiles that measure 2 inches on a side. $9 (8 \sqrt{2}) + 8 (2 \sqrt{2})$ . Determine the width of the accent strip by simplifying the expression $9 (8 \sqrt{2}) + 8 (2 \sqrt{2})$ . (Round to the nearest tenth of an inch.)

230.

Suzy wants to use square tiles on the border of a spa she is installing in her backyard. She will use large tiles that have area of 12 square inches, medium tiles that have area of 8 square inches, and small tiles that have area of 4 square inches. Once section of the border will require 4 large tiles, 8 medium tiles, and 10 small tiles to cover the width of the wall. Simplify the expression $4 \sqrt{12} + 8 \sqrt{8} + 10 \sqrt{4}$ to determine the width of the wall.

Writing Exercises

231.

Explain the difference between like radicals and unlike radicals. Make sure your answer makes sense for radicals containing both numbers and variables.

232.

Explain the process for determining whether two radicals are like or unlike. Make sure your answer makes sense for radicals containing both numbers and variables.

Self Check

ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

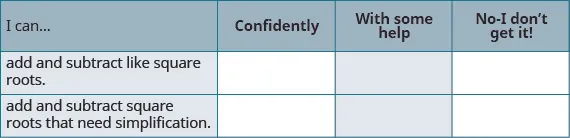
ⓑ What does this checklist tell you about your mastery of this section? What steps will you take to improve?