Jay Abramson

Learning Objectives

In this section, you will:

Identify nondegenerate conic sections given their general form equations.
Use rotation of axes formulas.
Write equations of rotated conics in standard form.
Identify conics without rotating axes.

As we have seen, conic sections are formed when a plane intersects two right circular cones aligned tip to tip and extending infinitely far in opposite directions, which we also call a cone. The way in which we slice the cone will determine the type of conic section formed at the intersection. A circle is formed by slicing a cone with a plane perpendicular to the axis of symmetry of the cone. An ellipse is formed by slicing a single cone with a slanted plane not perpendicular to the axis of symmetry. A parabola is formed by slicing the plane through the top or bottom of the double-cone, whereas a hyperbola is formed when the plane slices both the top and bottom of the cone. See Figure 1.

Figure 1 The nondegenerate conic sections

Ellipses, circles, hyperbolas, and parabolas are sometimes called the nondegenerate conic sections, in contrast to the degenerate conic sections, which are shown in Figure 2. A degenerate conic results when a plane intersects the double cone and passes through the apex. Depending on the angle of the plane, three types of degenerate conic sections are possible: a point, a line, or two intersecting lines.

Figure 2 Degenerate conic sections

Identifying Nondegenerate Conics in General Form

In previous sections of this chapter, we have focused on the standard form equations for nondegenerate conic sections. In this section, we will shift our focus to the general form equation, which can be used for any conic. The general form is set equal to zero, and the terms and coefficients are given in a particular order, as shown below.

A x^{2} + B x y + C y^{2} + D x + E y + F = 0

where $A, B,$ and $C$ are not all zero. We can use the values of the coefficients to identify which type conic is represented by a given equation.

You may notice that the general form equation has an $x y$ term that we have not seen in any of the standard form equations. As we will discuss later, the $x y$ term rotates the conic whenever $B$ is not equal to zero.

Conic Sections	Example
ellipse	$4 x^{2} + 9 y^{2} = 1$
circle	$4 x^{2} + 4 y^{2} = 1$
hyperbola	$4 x^{2} - 9 y^{2} = 1$
parabola	$4 x^{2} = 9 y or 4 y^{2} = 9 x$
one line	$4 x + 9 y = 1$
intersecting lines	$(x - 4) (y + 4) = 0$
parallel lines	$(x - 4) (x - 9) = 0$
a point	$4 x^{2} + 4 y^{2} = 0$
no graph	$4 x^{2} + 4 y^{2} = - 1$

Table 1

General Form of Conic Sections

A conic section has the general form

A x^{2} + B x y + C y^{2} + D x + E y + F = 0

where $A, B,$ and $C$ are not all zero.

Table 2 summarizes the different conic sections where $B = 0,$ and $A$ and $C$ are nonzero real numbers. This indicates that the conic has not been rotated.

ellipse	$A x^{2} + C y^{2} + D x + E y + F = 0, A \neq C and A C > 0$
circle	$A x^{2} + C y^{2} + D x + E y + F = 0, A = C$
hyperbola	$A x^{2} - C y^{2} + D x + E y + F = 0 or - A x^{2} + C y^{2} + D x + E y + F = 0,$ where $A$ and $C$ are positive
parabola	$A x^{2} + D x + E y + F = 0 or C y^{2} + D x + E y + F = 0$

Table 2

How To

Given the equation of a conic, identify the type of conic.

Rewrite the equation in the general form, $A x^{2} + B x y + C y^{2} + D x + E y + F = 0.$
Identify the values of A A and C C from the general form.
1. If $A$ and $C$ are nonzero, have the same sign, and are not equal to each other, then the graph may be an ellipse.
2. If $A$ and $C$ are equal and nonzero and have the same sign, then the graph may be a circle.
3. If $A$ and $C$ are nonzero and have opposite signs, then the graph may be a hyperbola.
4. If either $A$ or $C$ is zero, then the graph may be a parabola.
If B = 0, the conic section will have a vertical and/or horizontal axes. If B does not equal 0, as shown below, the conic section is rotated. Notice the phrase “may be” in the definitions. That is because the equation may not represent a conic section at all, depending on the values of A, B, C, D, E, and F. For example, the degenerate case of a circle or an ellipse is a point:
$A x^{2} + B y^{2} = 0,$ when A and B have the same sign.
The degenerate case of a hyperbola is two intersecting straight lines: $A x^{2} + B y^{2} = 0,$ when A and B have opposite signs.
On the other hand, the equation, $A x^{2} + B y^{2} + 1 = 0,$ when A and B are positive does not represent a graph at all, since there are no real ordered pairs which satisfy it.

Example 1

Identifying a Conic from Its General Form

Identify the graph of each of the following nondegenerate conic sections.

ⓐ $4 x^{2} - 9 y^{2} + 36 x + 36 y - 125 = 0$
ⓑ $9 y^{2} + 16 x + 36 y - 10 = 0$
ⓒ $3 x^{2} + 3 y^{2} - 2 x - 6 y - 4 = 0$
ⓓ $- 25 x^{2} - 4 y^{2} + 100 x + 16 y + 20 = 0$

Solution

ⓐ Rewriting the general form, we have
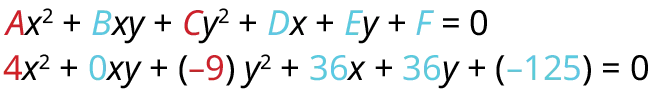
$A = 4$ and $C = −9,$ so we observe that $A$ and $C$ have opposite signs. The graph of this equation is a hyperbola.
ⓑ Rewriting the general form, we have
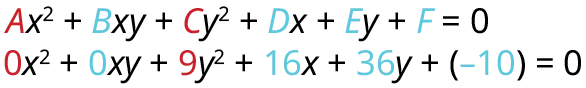
$A = 0$ and $C = 9.$ We can determine that the equation is a parabola, since $A$ is zero.
ⓒ Rewriting the general form, we have
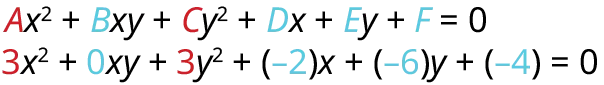
$A = 3$ and $C = 3.$ Because $A = C,$ the graph of this equation is a circle.
ⓓ Rewriting the general form, we have
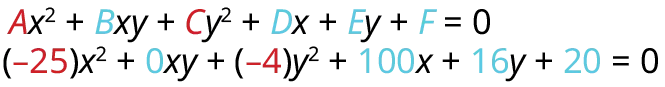
$A = −25$ and $C = −4.$ Because $A C > 0$ and $A \neq C,$ the graph of this equation is an ellipse.

Try It #1

Identify the graph of each of the following nondegenerate conic sections.

ⓐ $16 y^{2} - x^{2} + x - 4 y - 9 = 0$
ⓑ $16 x^{2} + 4 y^{2} + 16 x + 49 y - 81 = 0$

Finding a New Representation of the Given Equation after Rotating through a Given Angle

Until now, we have looked at equations of conic sections without an $x y$ term, which aligns the graphs with the x- and y-axes. When we add an $x y$ term, we are rotating the conic about the origin. If the x- and y-axes are rotated through an angle, say $θ,$ then every point on the plane may be thought of as having two representations: $(x, y)$ on the Cartesian plane with the original x-axis and y-axis, and $(x^{'}, y^{'})$ on the new plane defined by the new, rotated axes, called the x'-axis and y'-axis. See Figure 3.

Figure 3 The graph of the rotated ellipse

x^{2} + y^{2} - x y - 15 = 0

We will find the relationships between $x$ and $y$ on the Cartesian plane with $x^{'}$ and $y^{'}$ on the new rotated plane. See Figure 4.

Figure 4 The Cartesian plane with x- and y-axes and the resulting x′− and y′−axes formed by a rotation by an angle

θ .

The original coordinate x- and y-axes have unit vectors $i$ and $j .$ The rotated coordinate axes have unit vectors $i^{'}$ and $j^{'} .$ The angle $θ$ is known as the angle of rotation. See Figure 5. We may write the new unit vectors in terms of the original ones.

\begin{array}{l} i^{'} = \cos θ i + \sin θ j \\ j^{'} = - \sin θ i + \cos θ j \end{array}

Figure 5 Relationship between the old and new coordinate planes.

Consider a vector $u$ in the new coordinate plane. It may be represented in terms of its coordinate axes.

\begin{array}{l} u = x^{'} i^{'} + y^{'} j^{'} \\ u = x^{'} (i \cos θ + j \sin θ) + y^{'} (- i \sin θ + j \cos θ) & \begin{matrix}  \end{matrix} Substitute . \\ u = i x' \cos θ + j x' \sin θ - i y' \sin θ + j y' \cos θ & \begin{matrix}  \end{matrix} Distribute . \\ u = i x' \cos θ - i y' \sin θ + j x' \sin θ + j y' \cos θ & \begin{matrix}  \end{matrix} Apply commutative property . \\ u = (x' \cos θ - y' \sin θ) i + (x' \sin θ + y' \cos θ) j & \begin{matrix}  \end{matrix} Factor by grouping . \end{array}

Because $u = x^{'} i^{'} + y^{'} j^{'},$ we have representations of $x$ and $y$ in terms of the new coordinate system.

\begin{matrix} x = x^{'} \cos θ - y^{'} \sin θ \\ and \\ y = x^{'} \sin θ + y^{'} \cos θ \end{matrix}

Equations of Rotation

If a point $(x, y)$ on the Cartesian plane is represented on a new coordinate plane where the axes of rotation are formed by rotating an angle $θ$ from the positive x-axis, then the coordinates of the point with respect to the new axes are $(x^{'}, y^{'}) .$ We can use the following equations of rotation to define the relationship between $(x, y)$ and $(x^{'}, y^{'}) :$

x = x^{'} \cos θ - y^{'} \sin θ

and

y = x^{'} \sin θ + y^{'} \cos θ

How To

Given the equation of a conic, find a new representation after rotating through an angle.

Find $x$ and $y$ where $x = x^{'} \cos θ - y^{'} \sin θ$ and $y = x^{'} \sin θ + y^{'} \cos θ .$
Substitute the expression for $x$ and $y$ into in the given equation, then simplify.
Write the equations with $x^{'}$ and $y^{'}$ in standard form.

Example 2

Finding a New Representation of an Equation after Rotating through a Given Angle

Find a new representation of the equation $2 x^{2} - x y + 2 y^{2} - 30 = 0$ after rotating through an angle of $θ = 45° .$

Solution

Find $x$ and $y,$ where $x = x^{'} \cos θ - y^{'} \sin θ$ and $y = x^{'} \sin θ + y^{'} \cos θ .$

Because $θ = 45°,$

\begin{array}{l} x = x^{'} \cos (45°) - y^{'} \sin (45°) \\ x = x^{'} (\frac{1}{\sqrt{2}}) - y^{'} (\frac{1}{\sqrt{2}}) \\ x = \frac{x^{'} - y^{'}}{\sqrt{2}} \end{array}

and

\begin{array}{l} \begin{array}{l} y = x^{'} \sin (45°) + y^{'} \cos (45°) \\ y = x^{'} (\frac{1}{\sqrt{2}}) + y^{'} (\frac{1}{\sqrt{2}}) \\ y = \frac{x^{'} + y^{'}}{\sqrt{2}} \end{array} \end{array}

Substitute $x = x^{'} \cos θ - y^{'} \sin θ$ and $y = x^{'} \sin θ + y^{'} \cos θ$ into $2 x^{2} - x y + 2 y^{2} - 30 = 0.$

2 {(\frac{x^{'} - y^{'}}{\sqrt{2}})}^{2} - (\frac{x^{'} - y^{'}}{\sqrt{2}}) (\frac{x^{'} + y^{'}}{\sqrt{2}}) + 2 {(\frac{x^{'} + y^{'}}{\sqrt{2}})}^{2} - 30 = 0

Simplify.

\begin{array}{l} 2 \frac{(x^{'} - y^{'}) (x^{'} - y^{'})}{2} - \frac{(x^{'} - y^{'}) (x^{'} + y^{'})}{2} + 2 \frac{(x^{'} + y^{'}) (x^{'} + y^{'})}{2} - 30 = 0 & \begin{matrix}  \end{matrix} FOIL method \\ x^{'}^{2} {- 2 x^{'} y}^{'} + y^{'}^{2} - \frac{(x^{'}^{2} - y^{'}^{2})}{2} + x^{'}^{2} + 2 x^{'} y^{'} + y^{'}^{2} - 30 = 0 & \begin{matrix}  \end{matrix} Combine like terms . \\ 2 x^{'}^{2} + 2 y^{'}^{2} - \frac{(x^{'}^{2} - y^{'}^{2})}{2} = 30 & \begin{matrix}  \end{matrix} Combine like terms . \\ 2 (2 x^{'}^{2} + 2 y^{'}^{2} - \frac{(x^{'}^{2} - y^{'}^{2})}{2}) = 2 (30) & \begin{matrix}  \end{matrix} Multiply both sides by 2 . \\ 4 x^{'}^{2} + 4 y^{'}^{2} - (x^{'}^{2} - y^{'}^{2}) = 60 & \begin{matrix}  \end{matrix} Simplify . \\ 4 x^{'}^{2} + 4 y^{'}^{2} - x^{'}^{2} + y^{'}^{2} = 60 & \begin{matrix}  \end{matrix} Distribute . \\ \frac{3 x^{'}^{2}}{60} + \frac{5 y^{'}^{2}}{60} = \frac{60}{60} & \begin{matrix}  \end{matrix} Set equal to 1 . \end{array}

Write the equations with $x^{'}$ and $y^{'}$ in the standard form.

\frac{{x^{'}}^{2}}{20} + \frac{{y^{'}}^{2}}{12} = 1

This equation is an ellipse. Figure 6 shows the graph.

Figure 6

Writing Equations of Rotated Conics in Standard Form

Now that we can find the standard form of a conic when we are given an angle of rotation, we will learn how to transform the equation of a conic given in the form $A x^{2} + B x y + C y^{2} + D x + E y + F = 0$ into standard form by rotating the axes. To do so, we will rewrite the general form as an equation in the $x^{'}$ and $y^{'}$ coordinate system without the $x^{'} y^{'}$ term, by rotating the axes by a measure of $θ$ that satisfies

\cot (2 θ) = \frac{A - C}{B}

We have learned already that any conic may be represented by the second degree equation

A x^{2} + B x y + C y^{2} + D x + E y + F = 0

where $A, B,$ and $C$ are not all zero. However, if $B \neq 0,$ then we have an $x y$ term that prevents us from rewriting the equation in standard form. To eliminate it, we can rotate the axes by an acute angle $θ$ where $\cot (2 θ) = \frac{A - C}{B} .$

If $\cot (2 θ) > 0,$ then $2 θ$ is in the first quadrant, and $θ$ is between $(0°, 45°) .$
If $\cot (2 θ) < 0,$ then $2 θ$ is in the second quadrant, and $θ$ is between $(45°, 90°) .$
If $A = C,$ then $θ = 45° .$

How To

Given an equation for a conic in the $x^{'} y^{'}$ system, rewrite the equation without the $x^{'} y^{'}$ term in terms of $x^{'}$ and $y^{'},$ where the $x^{'}$ and $y^{'}$ axes are rotations of the standard axes by $θ$ degrees.

Find $\cot (2 θ) .$
Find $\sin θ$ and $\cos θ .$
Substitute $\sin θ$ and $\cos θ$ into $x = x^{'} \cos θ - y^{'} \sin θ$ and $y = x^{'} \sin θ + y^{'} \cos θ .$
Substitute the expression for $x$ and $y$ into in the given equation, and then simplify.
Write the equations with $x^{'}$ and $y^{'}$ in the standard form with respect to the rotated axes.

Example 3

Rewriting an Equation with respect to the x′ and y′ axes without the x′y′ Term

Rewrite the equation $8 x^{2} - 12 x y + 17 y^{2} = 20$ in the $x^{'} y^{'}$ system without an $x^{'} y^{'}$ term.

Solution

First, we find $\cot (2 θ) .$ See Figure 7.

\begin{array}{l} 8 x^{2} - 12 x y + 17 y^{2} = 20 \Rightarrow A = 8, B = - 12 and C = 17 \\ \cot (2 θ) = \frac{A - C}{B} = \frac{8 - 17}{- 12} \\ \cot (2 θ) = \frac{- 9}{- 12} = \frac{3}{4} \end{array}

A right triangle in the first quadrant of the x y plane. The horizontal side is length 3 and is on the x-axis. The vertical side is length 4. The hypotenuse is length h and originates at the Origin. The acute angle at the origin is 2 theta.

Figure 7

\cot (2 θ) = \frac{3}{4} = \frac{adjacent}{opposite}

So the hypotenuse is

\begin{array}{r} 3^{2} + 4^{2} = h^{2} \\ 9 + 16 = h^{2} \\ 25 = h^{2} \\ h = 5 \end{array}

Next, we find $\sin θ$ and $\cos θ .$

\begin{array}{l} \begin{array}{l} \sin θ = \sqrt{\frac{1 - \cos (2 θ)}{2}} = \sqrt{\frac{1 - \frac{3}{5}}{2}} = \sqrt{\frac{\frac{5}{5} - \frac{3}{5}}{2}} = \sqrt{\frac{5 - 3}{5} \cdot \frac{1}{2}} = \sqrt{\frac{2}{10}} = \sqrt{\frac{1}{5}} \end{array} \\ \sin θ = \frac{1}{\sqrt{5}} \\ \cos θ = \sqrt{\frac{1 + \cos (2 θ)}{2}} = \sqrt{\frac{1 + \frac{3}{5}}{2}} = \sqrt{\frac{\frac{5}{5} + \frac{3}{5}}{2}} = \sqrt{\frac{5 + 3}{5} \cdot \frac{1}{2}} = \sqrt{\frac{8}{10}} = \sqrt{\frac{4}{5}} \\ \cos θ = \frac{2}{\sqrt{5}} \end{array}

Substitute the values of $\sin θ$ and $\cos θ$ into $x = x^{'} \cos θ - y^{'} \sin θ$ and $y = x^{'} \sin θ + y^{'} \cos θ .$

\begin{array}{l} \begin{array}{l} x = x^{'} \cos θ - y^{'} \sin θ \\ x = x^{'} (\frac{2}{\sqrt{5}}) - y^{'} (\frac{1}{\sqrt{5}}) \\ x = \frac{2 x^{'} - y^{'}}{\sqrt{5}} \end{array} \end{array}

and

\begin{array}{l} \begin{array}{l} y = x^{'} \sin θ + y^{'} \cos θ \end{array} \\ y = x^{'} (\frac{1}{\sqrt{5}}) + y^{'} (\frac{2}{\sqrt{5}}) \\ y = \frac{x^{'} + 2 y^{'}}{\sqrt{5}} \end{array}

Substitute the expressions for $x$ and $y$ into in the given equation, and then simplify.

\begin{array}{l} 8 {(\frac{2 x^{'} - y^{'}}{\sqrt{5}})}^{2} - 12 (\frac{2 x^{'} - y^{'}}{\sqrt{5}}) (\frac{x^{'} + 2 y^{'}}{\sqrt{5}}) + 17 {(\frac{x^{'} + 2 y^{'}}{\sqrt{5}})}^{2} = 20 \\ 8 (\frac{(2 x^{'} - y^{'}) (2 x^{'} - y^{'})}{5}) - 12 (\frac{(2 x^{'} - y^{'}) (x^{'} + 2 y^{'})}{5}) + 17 (\frac{(x^{'} + 2 y^{'}) (x^{'} + 2 y^{'})}{5}) = 20 \\ 8 (4 x^{'}^{2} - 4 x^{'} y^{'} + y^{'}^{2}) - 12 (2 x^{'}^{2} + 3 x^{'} y^{'} - 2 y^{'}^{2}) + 17 (x^{'}^{2} + 4 x^{'} y^{'} + 4 y^{'}^{2}) = 100 \\ 32 x^{'}^{2} - 32 x^{'} y^{'} + 8 y^{'}^{2} - 24 x^{'}^{2} - 36 x^{'} y^{'} + 24 y^{'}^{2} + 17 x^{'}^{2} + 68 x^{'} y^{'} + 68 y^{'}^{2} = 100 \\ 25 x^{'}^{2} + 100 y^{'}^{2} = 100 \\ \frac{25}{100} x^{'}^{2} + \frac{100}{100} y^{'}^{2} = \frac{100}{100} \end{array}

Write the equations with $x^{'}$ and $y^{'}$ in the standard form with respect to the new coordinate system.

\frac{{x^{'}}^{2}}{4} + \frac{{y^{'}}^{2}}{1} = 1

Figure 8 shows the graph of the ellipse.

Figure 8

Try It #2

Rewrite the $13 x^{2} - 6 \sqrt{3} x y + 7 y^{2} = 16$ in the $x^{'} y^{'}$ system without the $x^{'} y^{'}$ term.

Example 4

Graphing an Equation That Has No x′y′ Terms

Graph the following equation relative to the $x^{'} y^{'}$ system:

x^{2} + 12 x y - 4 y^{2} = 30

Solution

First, we find $\cot (2 θ) .$

x^{2} + 12 x y - 4 y^{2} = 20 \Rightarrow A = 1, B = 12, and C = −4

\begin{array}{l} \cot (2 θ) = \frac{A - C}{B} \\ \cot (2 θ) = \frac{1 - (−4)}{12} \\ \cot (2 θ) = \frac{5}{12} \end{array}

Because $\cot (2 θ) = \frac{5}{12},$ we can draw a reference triangle as in Figure 9.

A line with positive slope passing through the origin of the x y pane is shown. The x value of 5 is shown on the x-axis. The y value of 12 is shown on the y-axis. The angle the line makes with the x-axis is 2theta. The line is labeled cotangent (2 theta) = 5/12.

Figure 9

\cot (2 θ) = \frac{5}{12} = \frac{adjacent}{opposite}

Thus, the hypotenuse is

\begin{array}{r} 5^{2} + 12^{2} = h^{2} \\ 25 + 144 = h^{2} \\ 169 = h^{2} \\ h = 13 \end{array}

Next, we find $\sin θ$ and $\cos θ .$ We will use half-angle identities.

\begin{array}{l} \begin{array}{l} \sin θ = \sqrt{\frac{1 - \cos (2 θ)}{2}} = \sqrt{\frac{1 - \frac{5}{13}}{2}} = \sqrt{\frac{\frac{13}{13} - \frac{5}{13}}{2}} = \sqrt{\frac{8}{13} \cdot \frac{1}{2}} = \frac{2}{\sqrt{13}} \end{array} \\ \cos θ = \sqrt{\frac{1 + \cos (2 θ)}{2}} = \sqrt{\frac{1 + \frac{5}{13}}{2}} = \sqrt{\frac{\frac{13}{13} + \frac{5}{13}}{2}} = \sqrt{\frac{18}{13} \cdot \frac{1}{2}} = \frac{3}{\sqrt{13}} \end{array}

Now we find $x$ and $y .$

\begin{array}{l} x = x^{'} \cos θ - y^{'} \sin θ \\ x = x^{'} (\frac{3}{\sqrt{13}}) - y^{'} (\frac{2}{\sqrt{13}}) \\ x = \frac{3 x^{'} - 2 y^{'}}{\sqrt{13}} \end{array}

and

\begin{array}{l} y = x^{'} \sin θ + y^{'} \cos θ \\ y = x^{'} (\frac{2}{\sqrt{13}}) + y^{'} (\frac{3}{\sqrt{13}}) \\ y = \frac{2 x^{'} + 3 y^{'}}{\sqrt{13}} \end{array}

Now we substitute $x = \frac{3 x^{'} - 2 y^{'}}{\sqrt{13}}$ and $y = \frac{2 x^{'} + 3 y^{'}}{\sqrt{13}}$ into $x^{2} + 12 x y - 4 y^{2} = 30.$

\begin{array}{l} {(\frac{3 x^{'} - 2 y^{'}}{\sqrt{13}})}^{2} + 12 (\frac{3 x^{'} - 2 y^{'}}{\sqrt{13}}) (\frac{2 x^{'} + 3 y^{'}}{\sqrt{13}}) - 4 {(\frac{2 x^{'} + 3 y^{'}}{\sqrt{13}})}^{2} = 30 \\ (\frac{1}{13}) [{(3 x^{'} - 2 y^{'})}^{2} + 12 (3 x^{'} - 2 y^{'}) (2 x^{'} + 3 y^{'}) - 4 {(2 x^{'} + 3 y^{'})}^{2}] = 30 & Factor . \\ (\frac{1}{13}) [9 x^{'}^{2} - 12 x^{'} y^{'} + 4 y^{'}^{2} + 12 (6 x^{'}^{2} + 5 x^{'} y^{'} - 6 y^{'}^{2}) - 4 (4 x^{'}^{2} + 12 x^{'} y^{'} + 9 y^{'}^{2})] = 30 & Multiply . \\ (\frac{1}{13}) [9 x^{'}^{2} - 12 x^{'} y^{'} + 4 y^{'}^{2} + 72 x^{'}^{2} + 60 x^{'} y^{'} - 72 y^{'}^{2} - 16 x^{'}^{2} - 48 x^{'} y^{'} - 36 y^{'}^{2}] = 30 & Distribute . \\ (\frac{1}{13}) [65 x^{'}^{2} - 104 y^{'}^{2}] = 30 & Combine like terms . \\ 65 x^{'}^{2} - 104 y^{'}^{2} = 390 & Multiply . \\ \frac{x^{'}^{2}}{6} - \frac{4 y^{'}^{2}}{15} = 1 & Divide by 390 . \end{array}

Figure 10 shows the graph of the hyperbola $\frac{{x^{'}}^{2}}{6} - \frac{4 {y^{'}}^{2}}{15} = 1.$

Figure 10

Identifying Conics without Rotating Axes

Now we have come full circle. How do we identify the type of conic described by an equation? What happens when the axes are rotated? Recall, the general form of a conic is

A x^{2} + B x y + C y^{2} + D x + E y + F = 0

If we apply the rotation formulas to this equation we get the form

A^{'} {x^{'}}^{2} + B^{'} x^{'} y^{'} + C^{'} {y^{'}}^{2} + D^{'} x^{'} + E^{'} y^{'} + F^{'} = 0

It may be shown that $B^{2} - 4 A C = {B^{'}}^{2} - 4 A^{'} C^{'} .$ The expression does not vary after rotation, so we call the expression invariant. The discriminant, $B^{2} - 4 A C,$ is invariant and remains unchanged after rotation. Because the discriminant remains unchanged, observing the discriminant enables us to identify the conic section.

Using the Discriminant to Identify a Conic

If the equation $A x^{2} + B x y + C y^{2} + D x + E y + F = 0$ is transformed by rotating axes into the equation $A^{'} {x^{'}}^{2} + B^{'} x^{'} y^{'} + C^{'} {y^{'}}^{2} + D^{'} x^{'} + E^{'} y^{'} + F^{'} = 0,$ then $B^{2} - 4 A C = {B^{'}}^{2} - 4 A^{'} C^{'} .$

The equation $A x^{2} + B x y + C y^{2} + D x + E y + F = 0$ is an ellipse, a parabola, or a hyperbola, or a degenerate case of one of these.

If the discriminant, $B^{2} - 4 A C,$ is

$< 0,$ the conic section is an ellipse
$= 0,$ the conic section is a parabola
$> 0,$ the conic section is a hyperbola

Example 5

Identifying the Conic without Rotating Axes

Identify the conic for each of the following without rotating axes.

ⓐ $5 x^{2} + 2 \sqrt{3} x y + 2 y^{2} - 5 = 0$
ⓑ $5 x^{2} + 2 \sqrt{3} x y + 12 y^{2} - 5 = 0$

Solution

ⓐ Let’s begin by determining $A, B,$ and $C .$
$\underset{A}{\underset{︸}{5}} x^{2} + \underset{B}{\underset{︸}{2 \sqrt{3}}} x y + \underset{C}{\underset{︸}{2}} y^{2} - 5 = 0$

Now, we find the discriminant.

$\begin{array}{l} B^{2} - 4 A C = {(2 \sqrt{3})}^{2} - 4 (5) (2) \\ = 4 (3) - 40 \\ = 12 - 40 \\ = - 28 < 0 \end{array}$
Therefore, $5 x^{2} + 2 \sqrt{3} x y + 2 y^{2} - 5 = 0$ represents an ellipse.
ⓑ Again, let’s begin by determining $A, B,$ and $C .$
$\underset{A}{\underset{︸}{5}} x^{2} + \underset{B}{\underset{︸}{2 \sqrt{3}}} x y + \underset{C}{\underset{︸}{12}} y^{2} - 5 = 0$

Now, we find the discriminant.

$\begin{array}{l} B^{2} - 4 A C = {(2 \sqrt{3})}^{2} - 4 (5) (12) \\ = 4 (3) - 240 \\ = 12 - 240 \\ = - 228 < 0 \end{array}$
Therefore, $5 x^{2} + 2 \sqrt{3} x y + 12 y^{2} - 5 = 0$ represents an ellipse.

Try It #3

Identify the conic for each of the following without rotating axes.

ⓐ $x^{2} - 9 x y + 3 y^{2} - 12 = 0$
ⓑ $10 x^{2} - 9 x y + 4 y^{2} - 4 = 0$

Media

Access this online resource for additional instruction and practice with conic sections and rotation of axes.

Introduction to Conic Sections

12.4 Section Exercises

Verbal

1.

What effect does the $x y$ term have on the graph of a conic section?

2.

If the equation of a conic section is written in the form $A x^{2} + B y^{2} + C x + D y + E = 0$ and $A B = 0,$ what can we conclude?

3.

If the equation of a conic section is written in the form $A x^{2} + B x y + C y^{2} + D x + E y + F = 0,$ and $B^{2} - 4 A C > 0,$ what can we conclude?

4.

Given the equation $a x^{2} + 4 x + 3 y^{2} - 12 = 0,$ what can we conclude if $a > 0 ?$

5.

For the equation $A x^{2} + B x y + C y^{2} + D x + E y + F = 0,$ the value of $θ$ that satisfies $\cot (2 θ) = \frac{A - C}{B}$ gives us what information?

Algebraic

For the following exercises, determine which conic section is represented based on the given equation.

6.

$9 x^{2} + 4 y^{2} + 72 x + 36 y - 500 = 0$

7.

$x^{2} - 10 x + 4 y - 10 = 0$

8.

$2 x^{2} - 2 y^{2} + 4 x - 6 y - 2 = 0$

9.

$4 x^{2} - y^{2} + 8 x - 1 = 0$

10.

$4 y^{2} - 5 x + 9 y + 1 = 0$

11.

$2 x^{2} + 3 y^{2} - 8 x - 12 y + 2 = 0$

12.

$4 x^{2} + 9 x y + 4 y^{2} - 36 y - 125 = 0$

13.

$3 x^{2} + 6 x y + 3 y^{2} - 36 y - 125 = 0$

14.

$- 3 x^{2} + 3 \sqrt{3} x y - 4 y^{2} + 9 = 0$

15.

$2 x^{2} + 4 \sqrt{3} x y + 6 y^{2} - 6 x - 3 = 0$

16.

$- x^{2} + 4 \sqrt{2} x y + 2 y^{2} - 2 y + 1 = 0$

17.

$8 x^{2} + 4 \sqrt{2} x y + 4 y^{2} - 10 x + 1 = 0$

For the following exercises, find a new representation of the given equation after rotating through the given angle.

18.

$3 x^{2} + x y + 3 y^{2} - 5 = 0, θ = 45°$

19.

$4 x^{2} - x y + 4 y^{2} - 2 = 0, θ = 45°$

20.

$2 x^{2} + 8 x y - 1 = 0, θ = 30°$

21.

$- 2 x^{2} + 8 x y + 1 = 0, θ = 45°$

22.

$4 x^{2} + \sqrt{2} x y + 4 y^{2} + y + 2 = 0, θ = 45°$

For the following exercises, determine the angle $θ$ that will eliminate the $x y$ term and write the corresponding equation without the $x y$ term.

23.

$x^{2} + 3 \sqrt{3} x y + 4 y^{2} + y - 2 = 0$

24.

$4 x^{2} + 2 \sqrt{3} x y + 6 y^{2} + y - 2 = 0$

25.

$9 x^{2} - 3 \sqrt{3} x y + 6 y^{2} + 4 y - 3 = 0$

26.

$−3 x^{2} - \sqrt{3} x y - 2 y^{2} - x = 0$

27.

$16 x^{2} + 24 x y + 9 y^{2} + 6 x - 6 y + 2 = 0$

28.

$x^{2} + 4 x y + 4 y^{2} + 3 x - 2 = 0$

29.

$x^{2} + 4 x y + y^{2} - 2 x + 1 = 0$

30.

$4 x^{2} - 2 \sqrt{3} x y + 6 y^{2} - 1 = 0$

Graphical

For the following exercises, rotate through the given angle based on the given equation. Give the new equation and graph the original and rotated equation.

31.

$y = - x^{2}, θ = - 45^{\circ}$

32.

$x = y^{2}, θ = 45^{\circ}$

33.

$\frac{x^{2}}{4} + \frac{y^{2}}{1} = 1, θ = 45^{\circ}$

34.

$\frac{y^{2}}{16} + \frac{x^{2}}{9} = 1, θ = 45^{\circ}$

35.

$y^{2} - x^{2} = 1, θ = 45^{\circ}$

36.

$y = \frac{x^{2}}{2}, θ = 30^{\circ}$

37.

$x = {(y - 1)}^{2}, θ = 30^{\circ}$

38.

$\frac{x^{2}}{9} + \frac{y^{2}}{4} = 1, θ = 30^{\circ}$

For the following exercises, graph the equation relative to the $x^{'} y^{'}$ system in which the equation has no $x^{'} y^{'}$ term.

39.

$x y = 9$

40.

$x^{2} + 10 x y + y^{2} - 6 = 0$

41.

$x^{2} - 10 x y + y^{2} - 24 = 0$

42.

$4 x^{2} - 3 \sqrt{3} x y + y^{2} - 22 = 0$

43.

$6 x^{2} + 2 \sqrt{3} x y + 4 y^{2} - 21 = 0$

44.

$11 x^{2} + 10 \sqrt{3} x y + y^{2} - 64 = 0$

45.

$21 x^{2} + 2 \sqrt{3} x y + 19 y^{2} - 18 = 0$

46.

$16 x^{2} + 24 x y + 9 y^{2} - 130 x + 90 y = 0$

47.

$16 x^{2} + 24 x y + 9 y^{2} - 60 x + 80 y = 0$

48.

$13 x^{2} - 6 \sqrt{3} x y + 7 y^{2} - 16 = 0$

49.

$4 x^{2} - 4 x y + y^{2} - 8 \sqrt{5} x - 16 \sqrt{5} y = 0$

For the following exercises, determine the angle of rotation in order to eliminate the $x y$ term. Then graph the new set of axes.

50.

$6 x^{2} - 5 \sqrt{3} x y + y^{2} + 10 x - 12 y = 0$

51.

$6 x^{2} - 5 x y + 6 y^{2} + 20 x - y = 0$

52.

$6 x^{2} - 8 \sqrt{3} x y + 14 y^{2} + 10 x - 3 y = 0$

53.

$4 x^{2} + 6 \sqrt{3} x y + 10 y^{2} + 20 x - 40 y = 0$

54.

$8 x^{2} + 3 x y + 4 y^{2} + 2 x - 4 = 0$

55.

$16 x^{2} + 24 x y + 9 y^{2} + 20 x - 44 y = 0$

For the following exercises, determine the value of $k$ based on the given equation.

56.

Given $4 x^{2} + k x y + 16 y^{2} + 8 x + 24 y - 48 = 0,$ find $k$ for the graph to be a parabola.

57.

Given $2 x^{2} + k x y + 12 y^{2} + 10 x - 16 y + 28 = 0,$ find $k$ for the graph to be an ellipse.

58.

Given $3 x^{2} + k x y + 4 y^{2} - 6 x + 20 y + 128 = 0,$ find $k$ for the graph to be a hyperbola.

59.

Given $k x^{2} + 8 x y + 8 y^{2} - 12 x + 16 y + 18 = 0,$ find $k$ for the graph to be a parabola.

60.

Given $6 x^{2} + 12 x y + k y^{2} + 16 x + 10 y + 4 = 0,$ find $k$ for the graph to be an ellipse.

12.4 Rotation of Axes

Identifying Nondegenerate Conics in General Form

Identifying a Conic from Its General Form

Solution

Finding a New Representation of the Given Equation after Rotating through a Given Angle

Finding a New Representation of an Equation after Rotating through a Given Angle

Solution

Writing Equations of Rotated Conics in Standard Form

Rewriting an Equation with respect to the x′ and y′ axes without the x′y′ Term

Solution

Graphing an Equation That Has No x′y′ Terms

Solution

Identifying Conics without Rotating Axes

Identifying the Conic without Rotating Axes

Solution

12.4 Section Exercises

Verbal

Algebraic

Graphical